Hints will display for most wrong answers; explanations for most right answers.   You can attempt a question multiple times; it will only be scored correct if you get it right the first time.

I used the official objectives and sample test to construct these questions, but cannot promise that they accurately reflect what’s on the real test.   Some of the sample questions were more convoluted than I could bear to write.   See terms of use.   See the MTEL Practice Test main page to view random questions on a variety of topics or to download paper practice tests.

MTEL General Curriculum Mathematics Practice

Question 1

Some children explored the diagonals in 2 x 2 squares on pages of a calendar (where all four squares have numbers in them).  They conjectured that the sum of the diagonals is always equal; in the example below, 8+16=9+15.

 

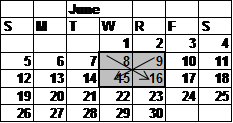
Which of the equations below could best be used to explain why the children's conjecture is correct?

A
\( \large 8x+16x=9x+15x\)
Hint:
What would x represent in this case? Make sure you can describe in words what x represents.
B
\( \large x+(x+2)=(x+1)+(x+1)\)
Hint:
What would x represent in this case? Make sure you can describe in words what x represents.
C
\( \large x+(x+8)=(x+1)+(x+7)\)
Hint:
x is the number in the top left square, x+8 is one below and to the right, x+1 is to the right of x, and x+7 is below x.
D
\( \large x+8+16=x+9+15\)
Hint:
What would x represent in this case? Make sure you can describe in words what x represents.
Question 1 Explanation: 
Topic: Recognize and apply the concepts of variable, equality, and equation to express relationships algebraically (Objective 0020).
Question 2

Here is a number trick:

 1) Pick a whole number

 2) Double your number.

 3) Add 20 to the above result.

 4) Multiply the above by 5

 5) Subtract 100

 6) Divide by 10

The result is always the number that you started with! Suppose you start by picking N. Which of the equations below best demonstrates that the result after Step 6 is also N?

A
\( \large N*2+20*5-100\div 10=N\)
Hint:
Use parentheses or else order of operations is off.
B
\( \large \left( \left( 2*N+20 \right)*5-100 \right)\div 10=N\)
C
\( \large \left( N+N+20 \right)*5-100\div 10=N\)
Hint:
With this answer you would subtract 10, instead of subtracting 100 and then dividing by 10.
D
\( \large \left( \left( \left( N\div 10 \right)-100 \right)*5+20 \right)*2=N\)
Hint:
This answer is quite backwards.
Question 2 Explanation: 
Topic: Recognize and apply the concepts of variable, function, equality, and equation to express relationships algebraically (Objective 0020).
Question 3

Use the problem below to answer the question that follows:

T shirts are on sale for 20% off. Tasha paid $8.73 for a shirt.  What is the regular price of the shirt? There is no tax on clothing purchases under $175.

Let p represent the regular price of these t-shirt. Which of the following equations is correct?

A
\( \large 0.8p=\$8.73\)
Hint:
80% of the regular price = $8.73.
B
\( \large \$8.73+0.2*\$8.73=p\)
Hint:
The 20% off was off of the ORIGINAL price, not off the $8.73 (a lot of people make this mistake). Plus this is the same equation as in choice c.
C
\( \large 1.2*\$8.73=p\)
Hint:
The 20% off was off of the ORIGINAL price, not off the $8.73 (a lot of people make this mistake). Plus this is the same equation as in choice b.
D
\( \large p-0.2*\$8.73=p\)
Hint:
Subtract p from both sides of this equation, and you have -.2 x 8.73 =0.
Question 3 Explanation: 
Topics: Use algebra to solve word problems involving percents and identify variables, and derive algebraic expressions that represent real-world situations (Objective 0020).
Question 4

Taxicab fares in Boston (Spring 2012) are $2.60 for the first \(\dfrac{1}{7}\) of a mile or less and $0.40 for each \(\dfrac{1}{7}\) of a mile after that.

Let d represent the distance a passenger travels in miles (with \(d>\dfrac{1}{7}\)). Which of the following expressions represents the total fare?

A
\( \large \$2.60+\$0.40d\)
Hint:
It's 40 cents for 1/7 of a mile, not per mile.
B
\( \large \$2.60+\$0.40\dfrac{d}{7}\)
Hint:
According to this equation, going 7 miles would cost $3; does that make sense?
C
\( \large \$2.20+\$2.80d\)
Hint:
You can think of the fare as $2.20 to enter the cab, and then $0.40 for each 1/7 of a mile, including the first 1/7 of a mile (or $2.80 per mile).

Alternatively, you pay $2.60 for the first 1/7 of a mile, and then $2.80 per mile for d-1/7 miles. The total is 2.60+2.80(d-1/7) = 2.60+ 2.80d -.40 = 2.20+2.80d.
D
\( \large \$2.60+\$2.80d\)
Hint:
Don't count the first 1/7 of a mile twice.
Question 4 Explanation: 
Topic: Identify variables and derive algebraic expressions that represent real-world situations (Objective 0020), and select the linear equation that best models a real-world situation (Objective 0022).
Question 5

Cell phone plan A charges $3 per month plus $0.10 per minute. Cell phone plan B charges $29.99 per month, with no fee for the first 400 minutes and then $0.20 for each additional minute.

Which equation can be used to solve for the number of minutes, m (with m>400) that a person would have to spend on the phone each month in order for the bills for plan A and plan B to be equal?

A
\( \large 3.10m=400+0.2m\)
Hint:
These are the numbers in the problem, but this equation doesn't make sense. If you don't know how to make an equation, try plugging in an easy number like m=500 minutes to see if each side equals what it should.
B
\( \large 3+0.1m=29.99+.20m\)
Hint:
Doesn't account for the 400 free minutes.
C
\( \large 3+0.1m=400+29.99+.20(m-400)\)
Hint:
Why would you add 400 minutes and $29.99? If you don't know how to make an equation, try plugging in an easy number like m=500 minutes to see if each side equals what it should.
D
\( \large 3+0.1m=29.99+.20(m-400)\)
Hint:
The left side is $3 plus $0.10 times the number of minutes. The right is $29.99 plus $0.20 times the number of minutes over 400.
Question 5 Explanation: 
Identify variables and derive algebraic expressions that represent real-world situations (Objective 0020).
Question 6

A sales companies pays its representatives $2 for each item sold, plus 40% of the price of the item.   The rest of the money that the representatives collect goes to the company.  All transactions are in cash, and all items cost $4 or more.   If the price of an item in dollars is p, which expression represents the amount of money the company collects when the item is sold?

A
\( \large \dfrac{3}{5}p-2\)
Hint:
The company gets 3/5=60% of the price, minus the $2 per item.
B
\( \large \dfrac{3}{5}\left( p-2 \right)\)
Hint:
This is sensible, but not what the problem states.
C
\( \large \dfrac{2}{5}p+2\)
Hint:
The company pays the extra $2; it doesn't collect it.
D
\( \large \dfrac{2}{5}p-2\)
Hint:
This has the company getting 2/5 = 40% of the price of each item, but that's what the representative gets.
Question 6 Explanation: 
Topic: Use algebra to solve word problems involving fractions, ratios, proportions, and percents (Objective 0020).
Question 7

Here is a student's work solving an equation:

\( x-4=-2x+6\)

\( x-4+4=-2x+6+4\)

\( x=-2x+10\)

\( x-2x=10\)

\( x=10\)

Which of the following statements is true?

A

The student‘s solution is correct.

Hint:
Try plugging into the original solution.
B

The student did not correctly use properties of equality.

Hint:
After \( x=-2x+10\), the student subtracted 2x on the left and added 2x on the right.
C

The student did not correctly use the distributive property.

Hint:
Distributive property is \(a(b+c)=ab+ac\).
D

The student did not correctly use the commutative property.

Hint:
Commutative property is \(a+b=b+a\) or \(ab=ba\).
Question 7 Explanation: 
Topic: Justify algebraic manipulations by application of the properties of equality, the order of operations, the number properties, and the order properties (Objective 0020).
Question 8

Solve for x: \(\large 4-\dfrac{2}{3}x=2x\)

A
\( \large x=3\)
Hint:
Try plugging x=3 into the equation.
B
\( \large x=-3\)
Hint:
Left side is positive, right side is negative when you plug this in for x.
C
\( \large x=\dfrac{3}{2}\)
Hint:
One way to solve: \(4=\dfrac{2}{3}x+2x\) \(=\dfrac{8}{3}x\).\(x=\dfrac{3 \times 4}{8}=\dfrac{3}{2}\). Another way is to just plug x=3/2 into the equation and see that each side equals 3 -- on a multiple choice test, you almost never have to actually solve for x.
D
\( \large x=-\dfrac{3}{2}\)
Hint:
Left side is positive, right side is negative when you plug this in for x.
Question 8 Explanation: 
Topic: Solve linear equations (Objective 0020).
Question 9

Which of the following is equivalent to

\( \large A-B+C\div D\times E\)?

A
\( \large A-B-\dfrac{C}{DE} \)
Hint:
In the order of operations, multiplication and division have the same priority, so do them left to right; same with addition and subtraction.
B
\( \large A-B+\dfrac{CE}{D}\)
Hint:
In practice, you're better off using parentheses than writing an expression like the one in the question. The PEMDAS acronym that many people memorize is misleading. Multiplication and division have equal priority and are done left to right. They have higher priority than addition and subtraction. Addition and subtraction also have equal priority and are done left to right.
C
\( \large \dfrac{AE-BE+CE}{D}\)
Hint:
Use order of operations, don't just compute left to right.
D
\( \large A-B+\dfrac{C}{DE}\)
Hint:
In the order of operations, multiplication and division have the same priority, so do them left to right
Question 9 Explanation: 
Topic: Justify algebraic manipulations by application of the properties of order of operations (Objective 0020).
Question 10

Use the solution procedure below to answer the question that follows:

\( \large {\left( x+3 \right)}^{2}=10\)

\( \large \left( x+3 \right)\left( x+3 \right)=10\)

\( \large {x}^{2}+9=10\)

\( \large {x}^{2}+9-9=10-9\)

\( \large {x}^{2}=1\)

\( \large x=1\text{ or }x=-1\)

Which of the following is incorrect in the procedure shown above?

A

The commutative property is used incorrectly.

Hint:
The commutative property is \(a+b=b+a\) or \(ab=ba\).
B

The associative property is used incorrectly.

Hint:
The associative property is \(a+(b+c)=(a+b)+c\) or \(a \times (b \times c)=(a \times b) \times c\).
C

Order of operations is done incorrectly.

D

The distributive property is used incorrectly.

Hint:
\((x+3)(x+3)=x(x+3)+3(x+3)\)=\(x^2+3x+3x+9.\)
Question 10 Explanation: 
Topic: Justify algebraic manipulations by application of the properties of equality, the order of operations, the number properties, and the order properties (Objective 0020).
There are 10 questions to complete.

If you found a mistake or have comments on a particular question, please contact me (please copy and paste at least part of the question into the form, as the numbers change depending on how quizzes are displayed).   General comments can be left here.