Hints will display for most wrong answers; explanations for most right answers. You can attempt a question multiple times; it will only be scored correct if you get it right the first time. To see five new questions, reload the page.
I used the official objectives and sample test to construct these questions, but cannot promise that they accurately reflect what’s on the real test. Some of the sample questions were more convoluted than I could bear to write. See terms of use. See the MTEL Practice Test main page to view questions on a particular topic or to download paper practice tests.
MTEL General Curriculum Mathematics Practice
Question 1 |
The expression \( \large {{7}^{-4}}\cdot {{8}^{-6}}\) is equal to which of the following?
\( \large \dfrac{8}{{{\left( 56 \right)}^{4}}}\) Hint: The bases are whole numbers, and the exponents are negative. How can the numerator be 8? | |
\( \large \dfrac{64}{{{\left( 56 \right)}^{4}}}\) Hint: The bases are whole numbers, and the exponents are negative. How can the numerator be 64? | |
\( \large \dfrac{1}{8\cdot {{\left( 56 \right)}^{4}}}\) Hint: \(8^{-6}=8^{-4} \times 8^{-2}\) | |
\( \large \dfrac{1}{64\cdot {{\left( 56 \right)}^{4}}}\) |
Question 2 |
The prime factorization of n can be written as n=pqr, where p, q, and r are distinct prime numbers. How many factors does n have, including 1 and itself?
\( \large3\) Hint: 1, p, q, r, and pqr are already 5, so this isn't enough. You might try plugging in p=2, q=3, and r=5 to help with this problem. | |
\( \large5\) Hint: Don't forget pq, etc. You might try plugging in p=2, q=3, and r=5 to help with this problem. | |
\( \large6\) Hint: You might try plugging in p=2, q=3, and r=5 to help with this problem. | |
\( \large8\) Hint: 1, p, q, r, pq, pr, qr, pqr. |
Question 3 |
In March of 2012, 1 dollar was worth the same as 0.761 Euros, and 1 dollar was also worth the same as 83.03 Japanese Yen. Which of the expressions below gives the number of Yen that are worth 1 Euro?
\( \large {83}.0{3}\cdot 0.{761}\) Hint: This equation gives less than the number of yen per dollar, but 1 Euro is worth more than 1 dollar. | |
\( \large \dfrac{0.{761}}{{83}.0{3}}\) Hint: Number is way too small. | |
\( \large \dfrac{{83}.0{3}}{0.{761}}\) Hint: One strategy here is to use easier numbers, say 1 dollar = .5 Euros and 100 yen, then 1 Euro would be 200 Yen (change the numbers in the equations and see what works). Another is to use dimensional analysis: we want # yen per Euro, or yen/Euro = yen/dollar \(\times\) dollar/Euro = \(83.03 \times \dfrac {1}{0.761}\) | |
\( \large \dfrac{1}{0.{761}}\cdot \dfrac{1}{{83}.0{3}}\) Hint: Number is way too small. |
Question 4 |
Below is a portion of a number line:
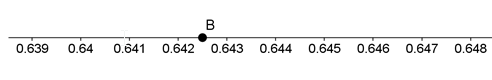
Point B is halfway between two tick marks. What number is represented by Point B?
\( \large 0.645\) Hint: That point is marked on the line, to the right. | |
\( \large 0.6421\) Hint: That point is to the left of point B. | |
\( \large 0.6422\) Hint: That point is to the left of point B. | |
\( \large 0.6425\) |
Question 5 |
The function d(x) gives the result when 12 is divided by x. Which of the following is a graph of d(x)?
 Hint: d(x) is 12 divided by x, not x divided by 12. | |
 Hint: When x=2, what should d(x) be? | |
 Hint: When x=2, what should d(x) be? | |
 |
If you found a mistake or have comments on a particular question, please contact me (please copy and paste at least part of the question into the form, as the numbers change depending on how quizzes are displayed). General comments can be left here.