Hints will display for most wrong answers; explanations for most right answers. You can attempt a question multiple times; it will only be scored correct if you get it right the first time.
I used the official objectives and sample test to construct these questions, but cannot promise that they accurately reflect what’s on the real test. Some of the sample questions were more convoluted than I could bear to write. See terms of use. See the MTEL Practice Test main page to view questions on a particular topic or to download paper practice tests.
MTEL General Curriculum Mathematics Practice
Question 1 |
The expression \( \large {{7}^{-4}}\cdot {{8}^{-6}}\) is equal to which of the following?
\( \large \dfrac{8}{{{\left( 56 \right)}^{4}}}\) Hint: The bases are whole numbers, and the exponents are negative. How can the numerator be 8? | |
\( \large \dfrac{64}{{{\left( 56 \right)}^{4}}}\) Hint: The bases are whole numbers, and the exponents are negative. How can the numerator be 64? | |
\( \large \dfrac{1}{8\cdot {{\left( 56 \right)}^{4}}}\) Hint: \(8^{-6}=8^{-4} \times 8^{-2}\) | |
\( \large \dfrac{1}{64\cdot {{\left( 56 \right)}^{4}}}\) |
Question 2 |
Use the expression below to answer the question that follows.
\( \large 3\times {{10}^{4}}+2.2\times {{10}^{2}}\)
Which of the following is closest to the expression above?
Five millionHint: Pay attention to the exponents. Adding 3 and 2 doesn't work because they have different place values. | |
Fifty thousandHint: Pay attention to the exponents. Adding 3 and 2 doesn't work because they have different place values. | |
Three millionHint: Don't add the exponents. | |
Thirty thousandHint: \( 3\times {{10}^{4}} = 30,000;\) the other term is much smaller and doesn't change the estimate. |
Question 3 |
What is the perimeter of a right triangle with legs of lengths x and 2x?
\( \large 6x\) Hint: Use the Pythagorean Theorem. | |
\( \large 3x+5{{x}^{2}}\) Hint: Don't forget to take square roots when you use the Pythagorean Theorem. | |
\( \large 3x+\sqrt{5}{{x}^{2}}\) Hint: \(\sqrt {5 x^2}\) is not \(\sqrt {5}x^2\). | |
\( \large 3x+\sqrt{5}{{x}^{{}}}\) Hint: To find the hypotenuse, h, use the Pythagorean Theorem: \(x^2+(2x)^2=h^2.\) \(5x^2=h^2,h=\sqrt{5}x\). The perimeter is this plus x plus 2x. |
Question 4 |
Below are front, side, and top views of a three-dimensional solid.
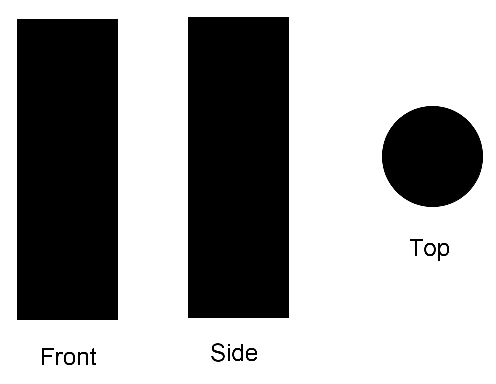
Which of the following could be the solid shown above?
A sphereHint: All views would be circles. | |
A cylinder | |
A coneHint: Two views would be triangles, not rectangles. | |
A pyramidHint: How would one view be a circle? |
Question 5 |
The pattern below consists of a row of black squares surrounded by white squares.
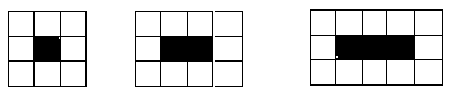
How many white squares would surround a row of 157 black squares?
314Hint: Try your procedure on a smaller number that you can count to see where you made a mistake. | |
317Hint: Are there ever an odd number of white squares? | |
320Hint: One way to see this is that there are 6 tiles on the left and right ends, and the rest of the white tiles are twice the number of black tiles (there are many other ways to look at it too). | |
322Hint: Try your procedure on a smaller number that you can count to see where you made a mistake. |
Question 6 |
The function d(x) gives the result when 12 is divided by x. Which of the following is a graph of d(x)?
 Hint: d(x) is 12 divided by x, not x divided by 12. | |
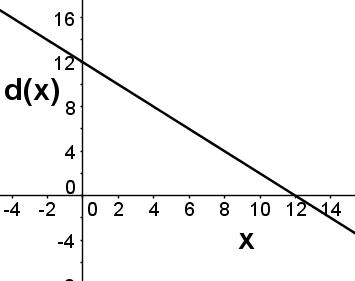 Hint: When x=2, what should d(x) be? | |
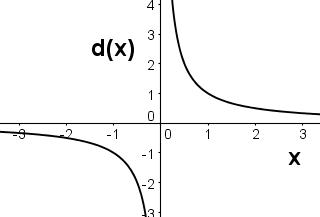 Hint: When x=2, what should d(x) be? | |
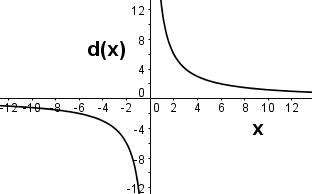 |
Question 7 |
The histogram below shows the frequency of a class's scores on a 4 question quiz.
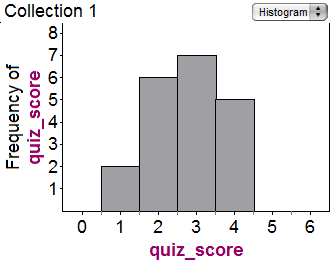
What was the mean score on the quiz?
\( \large 2.75\) Hint: There were 20 students who took the quiz. Total points earned: \(2 \times 1+6 \times 2+ 7\times 3+5 \times 4=55\), and 55/20 = 2.75. | |
\( \large 2\) Hint: How many students are there total? Did you count them all? | |
\( \large 3\) Hint: How many students are there total? Did you count them all? Be sure you're finding the mean, not the median or the mode. | |
\( \large 2.5\) Hint: How many students are there total? Did you count them all? Don't just take the mean of 1, 2, 3, 4 -- you have to weight them properly. |
Question 8 |
The table below gives data from various years on how many young girls drank milk.
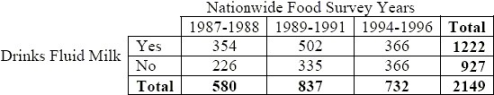
Based on the data given above, what was the probability that a randomly chosen girl in 1990 drank milk?
\( \large \dfrac{502}{1222}\) Hint: This is the probability that a randomly chosen girl who drinks milk was in the 1989-1991 food survey. | |
\( \large \dfrac{502}{2149}\) Hint: This is the probability that a randomly chosen girl from the whole survey drank milk and was also surveyed in 1989-1991. | |
\( \large \dfrac{502}{837}\) | |
\( \large \dfrac{1222}{2149}\) Hint: This is the probability that a randomly chosen girl from any year of the survey drank milk. |
Question 9 |
A cylindrical soup can has diameter 7 cm and height 11 cm. The can holds g grams of soup. How many grams of the same soup could a cylindrical can with diameter 14 cm and height 33 cm hold?
\( \large 6g\) Hint: You must scale in all three dimensions. | |
\( \large 12g\) Hint: Height is multiplied by 3, and diameter and radius are multiplied by 2. Since the radius is squared, final result is multiplied by \(2^2\times 3=12\). | |
\( \large 18g\) Hint: Don't square the height scale factor. | |
\( \large 36g\) Hint: Don't square the height scale factor. |
Question 10 |
At a school fundraising event, people can buy a ticket to spin a spinner like the one below. The region that the spinner lands in tells which, if any, prize the person wins.
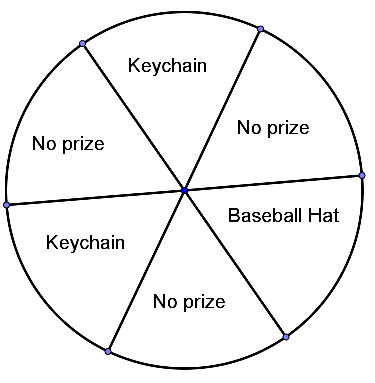
If 240 people buy tickets to spin the spinner, what is the best estimate of the number of keychains that will be given away?
40Hint: "Keychain" appears on the spinner twice. | |
80Hint: The probability of getting a keychain is 1/3, and so about 1/3 of the time the spinner will win. | |
100Hint: What is the probability of winning a keychain? | |
120Hint: That would be the answer for getting any prize, not a keychain specifically. |
Question 11 |
P is a prime number that divides 240. Which of the following must be true?
P divides 30Hint: 2, 3, and 5 are the prime factors of 240, and all divide 30. | |
P divides 48Hint: P=5 doesn't work. | |
P divides 75Hint: P=2 doesn't work. | |
P divides 80Hint: P=3 doesn't work. |
Question 12 |
Here is a student's work on several multiplication problems:
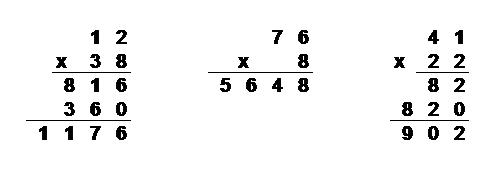 For which of the following problems is this student most likely to get the correct solution, even though he is using an incorrect algorithm?
For which of the following problems is this student most likely to get the correct solution, even though he is using an incorrect algorithm?
58 x 22Hint: This problem involves regrouping, which the student does not do correctly. | |
16 x 24Hint: This problem involves regrouping, which the student does not do correctly. | |
31 x 23Hint: There is no regrouping with this problem. | |
141 x 32Hint: This problem involves regrouping, which the student does not do correctly. |
Question 13 |
Which of the following is the equation of a linear function?
\( \large y={{x}^{2}}+2x+7\) Hint: This is a quadratic function. | |
\( \large y={{2}^{x}}\) Hint: This is an exponential function. | |
\( \large y=\dfrac{15}{x}\) Hint: This is an inverse function. | |
\( \large y=x+(x+4)\) Hint: This is a linear function, y=2x+4, it's graph is a straight line with slope 2 and y-intercept 4. |
Question 14 |
The "houses" below are made of toothpicks and gum drops.
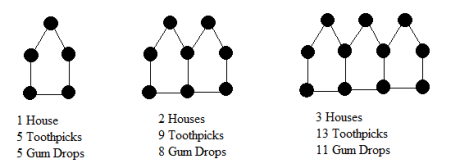
Which of the following does not represent the number of gumdrops in a row of h houses?
\( \large 2+3h\) Hint: Think of this as start with 2 gumdrops on the left wall, and then add 3 gumdrops for each house. | |
\( \large 5+3(h-1)\) Hint: Think of this as start with one house, and then add 3 gumdrops for each of the other h-1 houses. | |
\( \large h+(h+1)+(h+1)\) Hint: Look at the gumdrops in 3 rows: h gumdrops for the "rooftops," h+1 for the tops of the vertical walls, and h+1 for the floors. | |
\( \large 5+3h\) Hint: This one is not a correct equation (which makes it the correct answer!). Compare to choice A. One of them has to be wrong, as they differ by 3. |
Question 15 |
The Venn Diagram below gives data on the number of seniors, athletes, and vegetarians in the student body at a college:

How many students at the college are seniors who are not vegetarians?
\( \large 137\) Hint: Doesn't include the senior athletes who are not vegetarians. | |
\( \large 167\) | |
\( \large 197\) Hint: That's all seniors, including vegetarians. | |
\( \large 279\) Hint: Includes all athletes who are not vegetarians, some of whom are not seniors. |
Question 16 |
Which of the following is equivalent to
\( \large A-B+C\div D\times E\)?
\( \large A-B-\dfrac{C}{DE}
\) Hint: In the order of operations, multiplication and division have the same priority, so do them left to right; same with addition and subtraction. | |
\( \large A-B+\dfrac{CE}{D}\) Hint: In practice, you're better off using parentheses than writing an expression like the one in the question. The PEMDAS acronym that many people memorize is misleading. Multiplication and division have equal priority and are done left to right. They have higher priority than addition and subtraction. Addition and subtraction also have equal priority and are done left to right. | |
\( \large \dfrac{AE-BE+CE}{D}\) Hint: Use order of operations, don't just compute left to right. | |
\( \large A-B+\dfrac{C}{DE}\) Hint: In the order of operations, multiplication and division have the same priority, so do them left to right |
Question 17 |
In each expression below N represents a negative integer. Which expression could have a negative value?
\( \large {{N}^{2}}\) Hint: Squaring always gives a non-negative value. | |
\( \large 6-N\) Hint: A story problem for this expression is, if it was 6 degrees out at noon and N degrees out at sunrise, by how many degrees did the temperature rise by noon? Since N is negative, the answer to this question has to be positive, and more than 6. | |
\( \large -N\) Hint: If N is negative, then -N is positive | |
\( \large 6+N\) Hint: For example, if \(N=-10\), then \(6+N = -4\) |
Question 18 |
A family went on a long car trip. Below is a graph of how far they had driven at each hour.
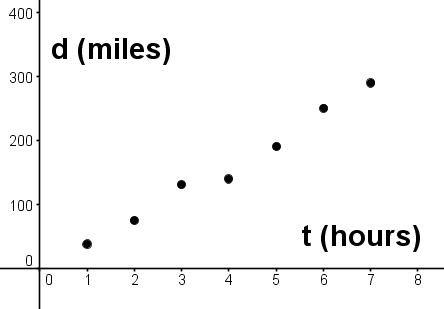
Which of the following is closest to their average speed driving on the trip?
\( \large d=20t\) Hint: Try plugging t=7 into the equation, and see how it matches the graph. | |
\( \large d=30t\) Hint: Try plugging t=7 into the equation, and see how it matches the graph. | |
\( \large d=40t\) | |
\( \large d=50t\) Hint: Try plugging t=7 into the equation, and see how it matches the graph. |
Question 19 |
Here is a mental math strategy for computing 26 x 16:
Step 1: 100 x 16 = 1600
Step 2: 25 x 16 = 1600 ÷· 4 = 400
Step 3: 26 x 16 = 400 + 16 = 416
Which property best justifies Step 3 in this strategy?
Commutative Property.Hint: For addition, the commutative property is \(a+b=b+a\) and for multiplication it's \( a \times b = b \times a\). | |
Associative Property.Hint: For addition, the associative property is \((a+b)+c=a+(b+c)\) and for multiplication it's \((a \times b) \times c=a \times (b \times c)\) | |
Identity Property.Hint: 0 is the additive identity, because \( a+0=a\) and 1 is the multiplicative identity because \(a \times 1=a\). The phrase "identity property" is not standard. | |
Distributive Property.Hint: \( (25+1) \times 16 = 25 \times 16 + 1 \times 16 \). This is an example of the distributive property of multiplication over addition. |
Question 20 |
A sales companies pays its representatives $2 for each item sold, plus 40% of the price of the item. The rest of the money that the representatives collect goes to the company. All transactions are in cash, and all items cost $4 or more. If the price of an item in dollars is p, which expression represents the amount of money the company collects when the item is sold?
\( \large \dfrac{3}{5}p-2\) Hint: The company gets 3/5=60% of the price, minus the $2 per item. | |
\( \large \dfrac{3}{5}\left( p-2 \right)\) Hint: This is sensible, but not what the problem states. | |
\( \large \dfrac{2}{5}p+2\) Hint: The company pays the extra $2; it doesn't collect it. | |
\( \large \dfrac{2}{5}p-2\) Hint: This has the company getting 2/5 = 40% of the price of each item, but that's what the representative gets. |
|
List |
If you found a mistake or have comments on a particular question, please contact me (please copy and paste at least part of the question into the form, as the numbers change depending on how quizzes are displayed). General comments can be left here.