Random Cool Math Thing #4

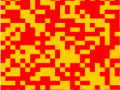
Visual Quadratic Reciprocity

This chart started with the odd primes less than 100 along the top and sides.  A square is red if the prime along the left is a perfect square modulo a prime along the top.

For example, if we look modulo 7, then

1²=1, 2²=4, 3²=2, 4²=2, 5²=4, 6²=1, and 7²=0, and after that the pattern repeats.  Hence, the only perfect squares modulo 7 are 0, 1, 2, and 4 (or other numbers congruent to them).  These perfect squares are called quadratic residues. The quadratic non-residues mod 7 are 3, 5, and 6.

The chart below shows how the top left corner of the image was constructed.  Looking at the column corresponding to mod 7, we see that the red -1’s correspond to the quadratic non-residues, 3 and 5, while the yellow 1’s correspond to the quadratic residues 7 (or 0) and 11 (or 4).

n\mod	3	5	7	11
3	1	-1	-1	1
5	-1	1	-1	1
7	1	-1	1	-1
11	-1	1	1	1

The Quadratic Reciprocity Theorem is related to symmetries in this table.  Notice that some entries, such as (3,5) and (5,3) are symmetric across the main diagonal, while others, such as (3,11) and (11,3) are not symmetric across the main diagonal.

Below is the table without the labels and numbers, just with the colors for odd primes less than 100.

Here is a small version with the odd primes below 200.

and a larger version of the same picture: