\(k^x-x^k\) Slider and \(x^y=y^x\) graph

Use the slider to change the value of k, and to see dynamically how the graph of \(h(x)=k^x-x^k\) changes. Negative values have \(x^k>k^x\). Which value of k gives a graph that is never negative? Why?

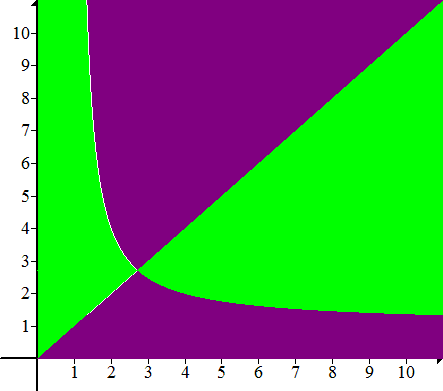
Here is a very pretty graph of \(x^y=y^x\) with areas where \(x^y < y^x\) shaded in green and areas where \(x^y > y^x\) shaded in purple.

I am working on an article about some problems related to these equations, including The Biggest Product Problem. There’s a lot of interesting stuff here!