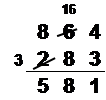Hints will display for most wrong answers; explanations for most right answers. You can attempt a question multiple times; it will only be scored correct if you get it right the first time.
I used the official objectives and sample test to construct these questions, but cannot promise that they accurately reflect what’s on the real test. Some of the sample questions were more convoluted than I could bear to write. See terms of use. See the MTEL Practice Test main page to view questions on a particular topic or to download paper practice tests.
MTEL General Curriculum Mathematics Practice
Question 1 |
How many lines of reflective symmetry and how many centers of rotational symmetry does the parallelogram depicted below have?

4 lines of reflective symmetry, 1 center of rotational symmetry.Hint: Try cutting out a shape like this one from paper, and fold where you think the lines of reflective symmetry are (or put a mirror there). Do things line up as you thought they would? | |
2 lines of reflective symmetry, 1 center of rotational symmetry.Hint: Try cutting out a shape like this one from paper, and fold where you think the lines of reflective symmetry are (or put a mirror there). Do things line up as you thought they would? | |
0 lines of reflective symmetry, 1 center of rotational symmetry.Hint: The intersection of the diagonals is a center of rotational symmetry. There are no lines of reflective symmetry, although many people get confused about this fact (best to play with hands on examples to get a feel). Just fyi, the letter S also has rotational, but not reflective symmetry, and it's one that kids often write backwards. | |
2 lines of reflective symmetry, 0 centers of rotational symmetry.Hint: Try cutting out a shape like this one from paper. Trace onto another sheet of paper. See if there's a way to rotate the cut out shape (less than a complete turn) so that it fits within the outlines again. |
Question 2 |
Exactly one of the numbers below is a prime number. Which one is it?
\( \large511 \) Hint: Divisible by 7. | |
\( \large517\) Hint: Divisible by 11. | |
\( \large519\) Hint: Divisible by 3. | |
\( \large521\) |
Question 3 |
A family on vacation drove the first 200 miles in 4 hours and the second 200 miles in 5 hours. Which expression below gives their average speed for the entire trip?
\( \large \dfrac{200+200}{4+5}\) Hint: Average speed is total distance divided by total time. | |
\( \large \left( \dfrac{200}{4}+\dfrac{200}{5} \right)\div 2\) Hint: This seems logical, but the problem is that it weights the first 4 hours and the second 5 hours equally, when each hour should get the same weight in computing the average speed. | |
\( \large \dfrac{200}{4}+\dfrac{200}{5} \) Hint: This would be an average of 90 miles per hour! | |
\( \large \dfrac{400}{4}+\dfrac{400}{5} \) Hint: This would be an average of 180 miles per hour! Even a family of race car drivers probably doesn't have that average speed on a vacation! |
Question 4 |
Which of the numbers below is the decimal equivalent of \( \dfrac{3}{8}?\)
0.38Hint: If you are just writing the numerator next to the denominator then your technique is way off, but by coincidence your answer is close; try with 2/3 and 0.23 is nowhere near correct. | |
0.125Hint: This is 1/8, not 3/8. | |
0.375 | |
0.83Hint: 3/8 is less than a half, and 0.83 is more than a half, so they can't be equal. |
Question 5 |
The following story situations model \( 12\div 3\):
I) Jack has 12 cookies, which he wants to share equally between himself and two friends. How many cookies does each person get?
II) Trent has 12 cookies, which he wants to put into bags of 3 cookies each. How many bags can he make?
III) Cicely has $12. Cookies cost $3 each. How many cookies can she buy?
Which of these questions illustrate the same model of division, either partitive (partioning) or measurement (quotative)?
I and II | |
I and III | |
II and IIIHint: Problem I is partitive (or partitioning or sharing) -- we put 12 objects into 3 groups. Problems II and III are quotative (or measurement) -- we put 12 objects in groups of 3. | |
All three problems model the same meaning of division |
Question 6 |
Below are front, side, and top views of a three-dimensional solid.
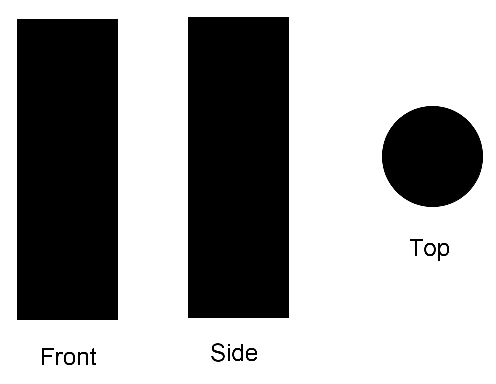
Which of the following could be the solid shown above?
A sphereHint: All views would be circles. | |
A cylinder | |
A coneHint: Two views would be triangles, not rectangles. | |
A pyramidHint: How would one view be a circle? |
Question 7 |
The window glass below has the shape of a semi-circle on top of a square, where the side of the square has length x. It was cut from one piece of glass.
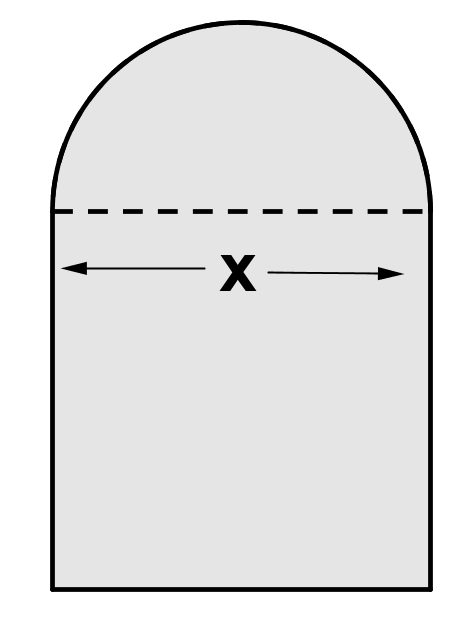
What is the perimeter of the window glass?
\( \large 3x+\dfrac{\pi x}{2}\) Hint: By definition, \(\pi\) is the ratio of the circumference of a circle to its diameter; thus the circumference is \(\pi d\). Since we have a semi-circle, its perimeter is \( \dfrac{1}{2} \pi x\). Only 3 sides of the square contribute to the perimeter. | |
\( \large 3x+2\pi x\) Hint: Make sure you know how to find the circumference of a circle. | |
\( \large 3x+\pi x\) Hint: Remember it's a semi-circle, not a circle. | |
\( \large 4x+2\pi x\) Hint: Only 3 sides of the square contribute to the perimeter. |
Question 8 |
Use the expression below to answer the question that follows:
\( \large \dfrac{\left( 7,154 \right)\times \left( 896 \right)}{216}\)
Which of the following is the best estimate of the expression above?
2,000Hint: The answer is bigger than 7,000. | |
20,000Hint: Estimate 896/216 first. | |
3,000Hint: The answer is bigger than 7,000. | |
30,000Hint: \( \dfrac{896}{216} \approx 4\) and \(7154 \times 4\) is over 28,000, so this answer is closest. |
Question 9 |
Use the samples of a student's work below to answer the question that follows:
This student divides fractions by first finding a common denominator, then dividing the numerators.
\( \large \dfrac{2}{3} \div \dfrac{3}{4} \longrightarrow \dfrac{8}{12} \div \dfrac{9}{12} \longrightarrow 8 \div 9 = \dfrac {8}{9}\) \( \large \dfrac{2}{5} \div \dfrac{7}{20} \longrightarrow \dfrac{8}{20} \div \dfrac{7}{20} \longrightarrow 8 \div 7 = \dfrac {8}{7}\) \( \large \dfrac{7}{6} \div \dfrac{3}{4} \longrightarrow \dfrac{14}{12} \div \dfrac{9}{12} \longrightarrow 14 \div 9 = \dfrac {14}{9}\)Which of the following best describes the mathematical validity of the algorithm the student is using?
It is not valid. Common denominators are for adding and subtracting fractions, not for dividing them.Hint: Don't be so rigid! Usually there's more than one way to do something in math. | |
It got the right answer in these three cases, but it isn‘t valid for all rational numbers.Hint: Did you try some other examples? What makes you say it's not valid? | |
It is valid if the rational numbers in the division problem are in lowest terms and the divisor is not zero.Hint: Lowest terms doesn't affect this problem at all. | |
It is valid for all rational numbers, as long as the divisor is not zero.Hint: When we have common denominators, the problem is in the form a/b divided by c/b, and the answer is a/c, as the student's algorithm predicts. |
Question 10 |
Use the graph below to answer the question that follows:
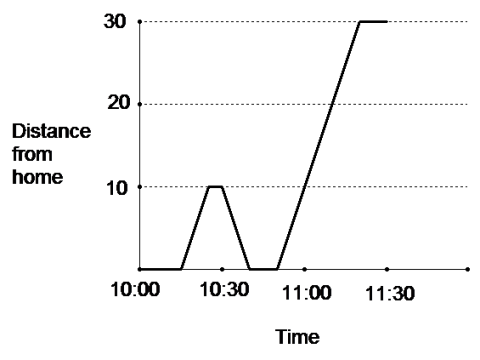
The graph above best matches which of the following scenarios:
George left home at 10:00 and drove to work on a crooked path. He was stopped in traffic at 10:30 and 10:45. He drove 30 miles total.Hint: Just because he ended up 30 miles from home doesn't mean he drove 30 miles total. | |
George drove to work. On the way to work there is a little hill and a big hill. He slowed down for them. He made it to work at 11:15.Hint: The graph is not a picture of the roads. | |
George left home at 10:15. He drove 10 miles, then realized he‘d forgotten something at home. He turned back and got what he‘d forgotten. Then he drove in a straight line, at many different speeds, until he got to work around 11:15.Hint: A straight line on a distance versus time graph means constant speed. | |
George left home at 10:15. He drove 10 miles, then realized he‘d forgotten something at home. He turned back and got what he‘d forgotten. Then he drove at a constant speed until he got to work around 11:15. |
Question 11 |
The chart below gives percentiles for the number of sit-ups that boys of various ages can do in 60 seconds (source , June 24, 2011)
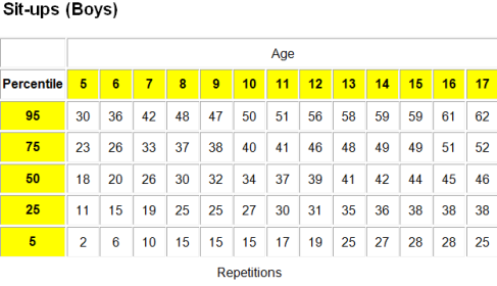
Which of the following statements can be inferred from the above chart?
95% of 12 year old boys can do 56 sit-ups in 60 seconds.Hint: The 95th percentile means that 95% of scores are less than or equal to 56, and 5% are greater than or equal to 56. | |
At most 25% of 7 year old boys can do 19 or more sit-ups in 60 seconds.Hint: The 25th percentile means that 25% of scores are less than or equal to 19, and 75% are greater than or equal to 19. | |
Half of all 13 year old boys can do less than 41 sit-ups in 60 seconds and half can do more than 41 sit-ups in 60 seconds.Hint: Close, but not quite. There's no accounting for boys who can do exactly 41 sit ups. Look at these data: 10, 20, 41, 41, 41, 41, 50, 60, 90. The median is 41, but more than half can do 41 or more. | |
At least 75% of 16 year old boys can only do 51 or fewer sit-ups in 60 seconds.Hint: The "at least" is necessary due to duplicates. Suppose the data were 10, 20, 51, 51. The 75th percentile is 51, but 100% of the boys can only do 51 or fewer situps. |
Question 12 |
Which of the graphs below represent functions?
I.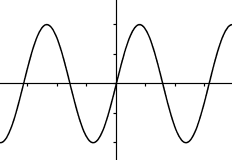 II.
II. 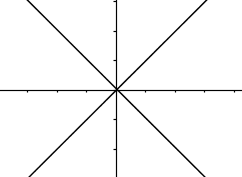 III.
III. 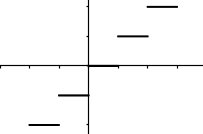 IV.
IV. 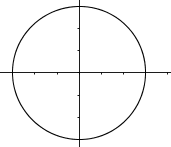
I and IV only.Hint: There are vertical lines that go through 2 points in IV . | |
I and III only.Hint: Even though III is not continuous, it's still a function (assuming that vertical lines between the "steps" do not go through 2 points). | |
II and III only.Hint: Learn about the vertical line test. | |
I, II, and IV only.Hint: There are vertical lines that go through 2 points in II. |
Question 13 |
The equation \( \large F=\frac{9}{5}C+32\) is used to convert a temperature measured in Celsius to the equivalent Farentheit temperature.
A patient's temperature increased by 1.5° Celcius. By how many degrees Fahrenheit did her temperature increase?
1.5°Hint: Celsius and Fahrenheit don't increase at the same rate. | |
1.8°Hint: That's how much the Fahrenheit temp increases when the Celsius temp goes up by 1 degree. | |
2.7°Hint: Each degree increase in Celsius corresponds to a \(\dfrac{9}{5}=1.8\) degree increase in Fahrenheit. Thus the increase is 1.8+0.9=2.7. | |
Not enough information.Hint: A linear equation has constant slope, which means that every increase of the same amount in one variable, gives a constant increase in the other variable. It doesn't matter what temperature the patient started out at. |
Question 14 |
Aya and Kendra want to estimate the height of a tree. On a sunny day, Aya measures Kendra's shadow as 3 meters long, and Kendra measures the tree's shadow as 15 meters long. Kendra is 1.5 meters tall. How tall is the tree?
7.5 metersHint: Here is a picture, note that the large and small right triangles are similar:  One way to do the problem is to note that there is a dilation (scale) factor of 5 on the shadows, so there must be that factor on the heights too. Another way is to note that the shadows are twice as long as the heights. | |
22.5 metersHint: Draw a picture. | |
30 metersHint: Draw a picture. | |
45 metersHint: Draw a picture. |
Question 15 |
A car is traveling at 60 miles per hour. Which of the expressions below could be used to compute how many feet the car travels in 1 second? Note that 1 mile = 5,280 feet.
\( \large 60\dfrac{\text{miles}}{\text{hour}}\cdot 5280\dfrac{\text{feet}}{\text{mile}}\cdot 60\dfrac{\text{minutes}}{\text{hour}}\cdot 60\dfrac{\text{seconds}}{\text{minute}}
\) Hint: This answer is not in feet/second. | |
\( \large 60\dfrac{\text{miles}}{\text{hour}}\cdot 5280\dfrac{\text{feet}}{\text{mile}}\cdot \dfrac{1}{60}\dfrac{\text{hour}}{\text{minutes}}\cdot \dfrac{1}{60}\dfrac{\text{minute}}{\text{seconds}}
\) Hint: This is the only choice where the answer is in feet per second and the unit conversions are correct. | |
\( \large 60\dfrac{\text{miles}}{\text{hour}}\cdot \dfrac{1}{5280}\dfrac{\text{foot}}{\text{miles}}\cdot 60\dfrac{\text{hours}}{\text{minute}}\cdot \dfrac{1}{60}\dfrac{\text{minute}}{\text{seconds}}\) Hint: Are there really 60 hours in a minute? | |
\( \large 60\dfrac{\text{miles}}{\text{hour}}\cdot \dfrac{1}{5280}\dfrac{\text{mile}}{\text{feet}}\cdot 60\dfrac{\text{minutes}}{\text{hour}}\cdot \dfrac{1}{60}\dfrac{\text{minute}}{\text{seconds}}\) Hint: This answer is not in feet/second. |
Question 16 |
The expression \( \large{{8}^{3}}\cdot {{2}^{-10}}\) is equal to which of the following?
\( \large 2\) Hint: Write \(8^3\) as a power of 2. | |
\( \large \dfrac{1}{2}\) Hint: \(8^3 \cdot {2}^{-10}={(2^3)}^3 \cdot {2}^{-10}\) =\(2^9 \cdot {2}^{-10} =2^{-1}\) | |
\( \large 16\) Hint: Write \(8^3\) as a power of 2. | |
\( \large \dfrac{1}{16}\) Hint: Write \(8^3\) as a power of 2. |
Question 17 |
What is the length of side \(\overline{BD}\) in the triangle below, where \(\angle DBA\) is a right angle?
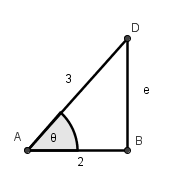
\( \large 1\) Hint: Use the Pythagorean Theorem. | |
\( \large \sqrt{5}\) Hint: \(2^2+e^2=3^2\) or \(4+e^2=9;e^2=5; e=\sqrt{5}\). | |
\( \large \sqrt{13}\) Hint: e is not the hypotenuse. | |
\( \large 5\) Hint: Use the Pythagorean Theorem. |
Question 18 |
Which of the following inequalities describes all values of x with \(\large \dfrac{x}{2}\le \dfrac{x}{3}\)?
\( \large x < 0\) Hint: If x =0, then x/2 = x/3, so this answer can't be correct. | |
\( \large x \le 0\) | |
\( \large x > 0\) Hint: If x =0, then x/2 = x/3, so this answer can't be correct. | |
\( \large x \ge 0\) Hint: Try plugging in x = 6. |
Question 19 |
Elena is going to use a calculator to check whether or not 267 is prime. She will pick certain divisors, and then find 267 divided by each, and see if she gets a whole number. If she never gets a whole number, then she's found a prime. Which numbers does Elena NEED to check before she can stop checking and be sure she has a prime?
All natural numbers from 2 to 266.Hint: She only needs to check primes -- checking the prime factors of any composite is enough to look for divisors. As a test taking strategy, the other three choices involve primes, so worth thinking about. | |
All primes from 2 to 266 .Hint: Remember, factors come in pairs (except for square root factors), so she would first find the smaller of the pair and wouldn't need to check the larger. | |
All primes from 2 to 133 .Hint: She doesn't need to check this high. Factors come in pairs, and something over 100 is going to be paired with something less than 3, so she will find that earlier. | |
All primes from \( \large 2\) to \( \large \sqrt{267}\).Hint: \(\sqrt{267} \times \sqrt{267}=267\). Any other pair of factors will have one factor less than \( \sqrt{267}\) and one greater, so she only needs to check up to \( \sqrt{267}\). |
Question 20 |
The picture below shows identical circles drawn on a piece of paper. The rectangle represents an index card that is blocking your view of \( \dfrac{3}{5}\) of the circles on the paper. How many circles are covered by the rectangle?

4Hint: The card blocks more than half of the circles, so this number is too small. | |
5Hint: The card blocks more than half of the circles, so this number is too small. | |
8Hint: The card blocks more than half of the circles, so this number is too small. | |
12Hint: 2/5 of the circles or 8 circles are showing. Thus 4 circles represent 1/5 of the circles, and \(4 \times 5=20\) circles represent 5/5 or all the circles. Thus 12 circles are hidden. |
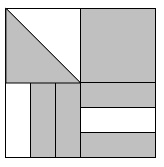
Question 21 |
Use the table below to answer the question that follows:
Gordon wants to buy three pounds of nuts. Each of the stores above ordinarily sells the nuts for $4.99 a pound, but is offering a discount this week. At which store can he buy the nuts for the least amount of money?
Store AHint: This would save about $2.50. You can quickly see that D saves more. | |
Store BHint: This saves 15% and C saves 25%. | |
Store C | |
Store DHint: This is about 20% off, which is less of a discount than C. |
Question 22 |
Here is a student's work solving an equation:
\( x-4=-2x+6\)
\( x-4+4=-2x+6+4\)
\( x=-2x+10\)
\( x-2x=10\)
\( x=10\)
Which of the following statements is true?
The student‘s solution is correct.Hint: Try plugging into the original solution. | |
The student did not correctly use properties of equality.Hint: After \( x=-2x+10\), the student subtracted 2x on the left and added 2x on the right. | |
The student did not correctly use the distributive property.Hint: Distributive property is \(a(b+c)=ab+ac\). | |
The student did not correctly use the commutative property.Hint: Commutative property is \(a+b=b+a\) or \(ab=ba\). |
Question 23 |
A homeowner is planning to tile the kitchen floor with tiles that measure 6 inches by 8 inches. The kitchen floor is a rectangle that measures 10 ft by 12 ft, and there are no gaps between the tiles. How many tiles does the homeowner need?
30Hint: The floor is 120 sq feet, and the tiles are smaller than 1 sq foot. Also, remember that 1 sq foot is 12 \(\times\) 12=144 sq inches. | |
120Hint: The floor is 120 sq feet, and the tiles are smaller than 1 sq foot. | |
300Hint: Recheck your calculations. | |
360Hint: One way to do this is to note that 6 inches = 1/2 foot and 8 inches = 2/3 foot, so the area of each tile is 1/2 \(\times\) 2/3=1/3 sq foot, or each square foot of floor requires 3 tiles. The area of the floor is 120 square feet. Note that the tiles would fit evenly oriented in either direction, parallel to the walls. |
Question 24 |
A teacher has a list of all the countries in the world and their populations in March 2012. She is going to have her students use technology to compute the mean and median of the numbers on the list. Which of the following statements is true?
The teacher can be sure that the mean and median will be the same without doing any computation.Hint: Does this make sense? How likely is it that the mean and median of any large data set will be the same? | |
The teacher can be sure that the mean is bigger than the median without doing any computation.Hint: This is a skewed distribution, and very large countries like China and India contribute huge numbers to the mean, but are counted the same as small countries like Luxembourg in the median (the same thing happens w/data on salaries, where a few very high income people tilt the mean -- that's why such data is usually reported as medians). | |
The teacher can be sure that the median is bigger than the mean without doing any computation.Hint: Think about a set of numbers like 1, 2, 3, 4, 10,000 -- how do the mean/median compare? How might that relate to countries of the world? | |
There is no way for the teacher to know the relative size of the mean and median without computing them.Hint: Knowing the shape of the distribution of populations does give us enough info to know the relative size of the mean and median, even without computing them. |
Question 25 |
Which property is not shared by all rhombi?
4 congruent sidesHint: The most common definition of a rhombus is a quadrilateral with 4 congruent sides. | |
A center of rotational symmetryHint: The diagonal of a rhombus separates it into two congruent isosceles triangles. The center of this line is a center of 180 degree rotational symmetry that switches the triangles. | |
4 congruent anglesHint: Unless the rhombus is a square, it does not have 4 congruent angles. | |
2 sets of parallel sidesHint: All rhombi are parallelograms. |
Question 26 |
Use the graph below to answer the question that follows:
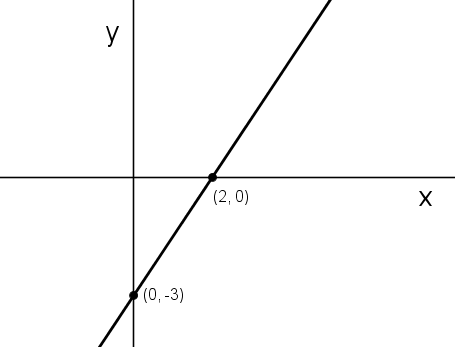
The graph above represents the equation \( \large 3x+Ay=B\), where A and B are integers. What are the values of A and B?
\( \large A = -2, B= 6\) Hint: Plug in (2,0) to get B=6, then plug in (0,-3) to get A=-2. | |
\( \large A = 2, B = 6\) Hint: Try plugging (0,-3) into this equation. | |
\( \large A = -1.5, B=-3\) Hint: The problem said that A and B were integers and -1.5 is not an integer. Don't try to use slope-intercept form. | |
\( \large A = 2, B = -3\) Hint: Try plugging (2,0) into this equation. |
Question 27 |
What is the perimeter of a right triangle with legs of lengths x and 2x?
\( \large 6x\) Hint: Use the Pythagorean Theorem. | |
\( \large 3x+5{{x}^{2}}\) Hint: Don't forget to take square roots when you use the Pythagorean Theorem. | |
\( \large 3x+\sqrt{5}{{x}^{2}}\) Hint: \(\sqrt {5 x^2}\) is not \(\sqrt {5}x^2\). | |
\( \large 3x+\sqrt{5}{{x}^{{}}}\) Hint: To find the hypotenuse, h, use the Pythagorean Theorem: \(x^2+(2x)^2=h^2.\) \(5x^2=h^2,h=\sqrt{5}x\). The perimeter is this plus x plus 2x. |
Question 28 |
The Americans with Disabilties Act (ADA) regulations state that the maximum slope for a wheelchair ramp in new construction is 1:12, although slopes between 1:16 and 1:20 are preferred. The maximum rise for any run is 30 inches. The graph below shows the rise and runs of four different wheelchair ramps. Which ramp is in compliance with the ADA regulations for new construction?
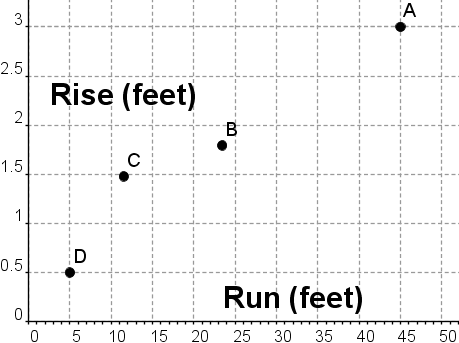
AHint: Rise is more than 30 inches. | |
BHint: Run is almost 24 feet, so rise can be almost 2 feet. | |
CHint: Run is 12 feet, so rise can be at most 1 foot. | |
DHint: Slope is 1:10 -- too steep. |
Question 29 |
Use the problem below to answer the question that follows:
T shirts are on sale for 20% off. Tasha paid $8.73 for a shirt. What is the regular price of the shirt? There is no tax on clothing purchases under $175.
Let p represent the regular price of these t-shirt. Which of the following equations is correct?
\( \large 0.8p=\$8.73\) Hint: 80% of the regular price = $8.73. | |
\( \large \$8.73+0.2*\$8.73=p\) Hint: The 20% off was off of the ORIGINAL price, not off the $8.73 (a lot of people make this mistake). Plus this is the same equation as in choice c. | |
\( \large 1.2*\$8.73=p\) Hint: The 20% off was off of the ORIGINAL price, not off the $8.73 (a lot of people make this mistake). Plus this is the same equation as in choice b. | |
\( \large p-0.2*\$8.73=p\) Hint: Subtract p from both sides of this equation, and you have -.2 x 8.73 =0. |
Question 30 |
The "houses" below are made of toothpicks and gum drops.
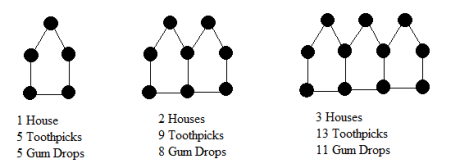
Which of the following does not represent the number of gumdrops in a row of h houses?
\( \large 2+3h\) Hint: Think of this as start with 2 gumdrops on the left wall, and then add 3 gumdrops for each house. | |
\( \large 5+3(h-1)\) Hint: Think of this as start with one house, and then add 3 gumdrops for each of the other h-1 houses. | |
\( \large h+(h+1)+(h+1)\) Hint: Look at the gumdrops in 3 rows: h gumdrops for the "rooftops," h+1 for the tops of the vertical walls, and h+1 for the floors. | |
\( \large 5+3h\) Hint: This one is not a correct equation (which makes it the correct answer!). Compare to choice A. One of them has to be wrong, as they differ by 3. |
Question 31 |
A family has four children. What is the probability that two children are girls and two are boys? Assume the the probability of having a boy (or a girl) is 50%.
\( \large \dfrac{1}{2}\) Hint: How many different configurations are there from oldest to youngest, e.g. BGGG? How many of them have 2 boys and 2 girls? | |
\( \large \dfrac{1}{4}\) Hint: How many different configurations are there from oldest to youngest, e.g. BGGG? How many of them have 2 boys and 2 girls? | |
\( \large \dfrac{1}{5}\) Hint: Some configurations are more probable than others -- i.e. it's more likely to have two boys and two girls than all boys. Be sure you are weighting properly. | |
\( \large \dfrac{3}{8}\) Hint: There are two possibilities for each child, so there are \(2 \times 2 \times 2 \times 2 =16\) different configurations, e.g. from oldest to youngest BBBG, BGGB, GBBB, etc. Of these configurations, there are 6 with two boys and two girls (this is the combination \(_{4}C_{2}\) or "4 choose 2"): BBGG, BGBG, BGGB, GGBB, GBGB, and GBBG. Thus the probability is 6/16=3/8. |
Question 32 |
A class is using base-ten block to represent numbers. A large cube represents 1000, a flat represents 100, a rod represents 10, and a little cube represents 1. Which of these is not a correct representation for 2,347?
23 flats, 4 rods, 7 little cubesHint: Be sure you read the question carefully: 2300+40+7=2347 | |
2 large cubes, 3 flats, 47 rodsHint: 2000+300+470 \( \neq\) 2347 | |
2 large cubes, 34 rods, 7 little cubesHint: Be sure you read the question carefully: 2000+340+7=2347 | |
2 large cubes, 3 flats, 4 rods, 7 little cubesHint: Be sure you read the question carefully: 2000+300+40+7=2347 |
Question 33 |
Which of the following is equivalent to
\( \large A-B+C\div D\times E\)?
\( \large A-B-\dfrac{C}{DE}
\) Hint: In the order of operations, multiplication and division have the same priority, so do them left to right; same with addition and subtraction. | |
\( \large A-B+\dfrac{CE}{D}\) Hint: In practice, you're better off using parentheses than writing an expression like the one in the question. The PEMDAS acronym that many people memorize is misleading. Multiplication and division have equal priority and are done left to right. They have higher priority than addition and subtraction. Addition and subtraction also have equal priority and are done left to right. | |
\( \large \dfrac{AE-BE+CE}{D}\) Hint: Use order of operations, don't just compute left to right. | |
\( \large A-B+\dfrac{C}{DE}\) Hint: In the order of operations, multiplication and division have the same priority, so do them left to right |
Question 34 |
The histogram below shows the frequency of a class's scores on a 4 question quiz.
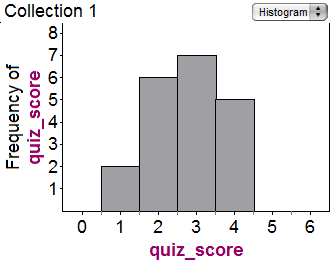
What was the mean score on the quiz?
\( \large 2.75\) Hint: There were 20 students who took the quiz. Total points earned: \(2 \times 1+6 \times 2+ 7\times 3+5 \times 4=55\), and 55/20 = 2.75. | |
\( \large 2\) Hint: How many students are there total? Did you count them all? | |
\( \large 3\) Hint: How many students are there total? Did you count them all? Be sure you're finding the mean, not the median or the mode. | |
\( \large 2.5\) Hint: How many students are there total? Did you count them all? Don't just take the mean of 1, 2, 3, 4 -- you have to weight them properly. |
Question 35 |
Which of the following sets of polygons can be assembled to form a pentagonal pyramid?
2 pentagons and 5 rectangles.Hint: These can be assembled to form a pentagonal prism, not a pentagonal pyramid. | |
1 square and 5 equilateral triangles.Hint: You need a pentagon for a pentagonal pyramid. | |
1 pentagon and 5 isosceles triangles. | |
1 pentagon and 10 isosceles triangles. |
Question 36 |
Here is a student's work on several multiplication problems:
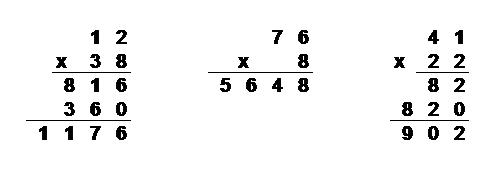 For which of the following problems is this student most likely to get the correct solution, even though he is using an incorrect algorithm?
For which of the following problems is this student most likely to get the correct solution, even though he is using an incorrect algorithm?
58 x 22Hint: This problem involves regrouping, which the student does not do correctly. | |
16 x 24Hint: This problem involves regrouping, which the student does not do correctly. | |
31 x 23Hint: There is no regrouping with this problem. | |
141 x 32Hint: This problem involves regrouping, which the student does not do correctly. |
Question 37 |
A solution requires 4 ml of saline for every 7 ml of medicine. How much saline would be required for 50 ml of medicine?
\( \large 28 \dfrac{4}{7}\) ml Hint: 49 ml of medicine requires 28 ml of saline. The extra ml of saline requires 4 ml saline/ 7 ml medicine = 4/7 ml saline per 1 ml medicine. | |
\( \large 28 \dfrac{1}{4}\) ml Hint: 49 ml of medicine requires 28 ml of saline. How much saline does the extra ml require? | |
\( \large 28 \dfrac{1}{7}\) ml Hint: 49 ml of medicine requires 28 ml of saline. How much saline does the extra ml require? | |
\( \large 87.5\) ml Hint: 49 ml of medicine requires 28 ml of saline. How much saline does the extra ml require? |
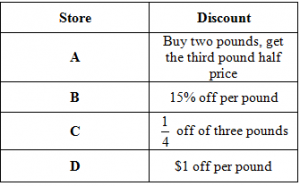
Question 38 |
\( \large \dfrac{17}{24}\) Hint: You might try adding segments so each quadrant is divided into 6 pieces with equal area -- there will be 24 regions, not all the same shape, but all the same area, with 17 of them shaded (for the top left quarter, you could also first change the diagonal line to a horizontal or vertical line that divides the square in two equal pieces and shade one) . | |
\( \large \dfrac{3}{4}\) Hint: Be sure you're taking into account the different sizes of the pieces. | |
\( \large \dfrac{2}{3}\) Hint: The bottom half of the picture is 2/3 shaded, and the top half is more than 2/3 shaded, so this answer is too small. | |
\( \large \dfrac{17}{6} \) Hint: This answer is bigger than 1, so doesn't make any sense. Be sure you are using the whole picture, not one quadrant, as the unit. |
Question 39 |
In January 2011, the national debt was about 14 trillion dollars and the US population was about 300 million people. Someone reading these figures estimated that the national debt was about $5,000 per person. Which of these statements best describes the reasonableness of this estimate?
It is too low by a factor of 10Hint: 14 trillion \( \approx 15 \times {{10}^{12}} \) and 300 million \( \approx 3 \times {{10}^{8}}\), so the true answer is about \( 5 \times {{10}^{4}} \) or $50,000. | |
It is too low by a factor of 100 | |
It is too high by a factor of 10 | |
It is too high by a factor of 100 |
Question 40 |
The speed of sound in dry air at 68 degrees F is 343.2 meters per second. Which of the expressions below could be used to compute the number of kilometers that a sound wave travels in 10 minutes (in dry air at 68 degrees F)?
\( \large 343.2\times 60\times 10\) Hint: In kilometers, not meters. | |
\( \large 343.2\times 60\times 10\times \dfrac{1}{1000}\) Hint: Units are meters/sec \(\times\) seconds/minute \(\times\) minutes \(\times\) kilometers/meter, and the answer is in kilometers. | |
\( \large 343.2\times \dfrac{1}{60}\times 10\) Hint: Include units and make sure answer is in kilometers. | |
\( \large 343.2\times \dfrac{1}{60}\times 10\times \dfrac{1}{1000}\) Hint: Include units and make sure answer is in kilometers. |
Question 41 |
Use the solution procedure below to answer the question that follows:
\( \large {\left( x+3 \right)}^{2}=10\)
\( \large \left( x+3 \right)\left( x+3 \right)=10\)
\( \large {x}^{2}+9=10\)
\( \large {x}^{2}+9-9=10-9\)
\( \large {x}^{2}=1\)
\( \large x=1\text{ or }x=-1\)
Which of the following is incorrect in the procedure shown above?
The commutative property is used incorrectly.Hint: The commutative property is \(a+b=b+a\) or \(ab=ba\). | |
The associative property is used incorrectly.Hint: The associative property is \(a+(b+c)=(a+b)+c\) or
\(a \times (b \times c)=(a \times b) \times c\). | |
Order of operations is done incorrectly. | |
The distributive property is used incorrectly.Hint: \((x+3)(x+3)=x(x+3)+3(x+3)\)=\(x^2+3x+3x+9.\) |
Question 42 |
Which of the following is the equation of a linear function?
\( \large y={{x}^{2}}+2x+7\) Hint: This is a quadratic function. | |
\( \large y={{2}^{x}}\) Hint: This is an exponential function. | |
\( \large y=\dfrac{15}{x}\) Hint: This is an inverse function. | |
\( \large y=x+(x+4)\) Hint: This is a linear function, y=2x+4, it's graph is a straight line with slope 2 and y-intercept 4. |
Question 43 |
A biology class requires a lab fee, which is a whole number of dollars, and the same amount for all students. On Monday the instructor collected $70 in fees, on Tuesday she collected $126, and on Wednesday she collected $266. What is the largest possible amount the fee could be?
$2Hint: A possible fee, but not the largest possible fee. Check the other choices to see which are factors of all three numbers. | |
$7Hint: A possible fee, but not the largest possible fee. Check the other choices to see which are factors of all three numbers. | |
$14Hint: This is the greatest common factor of 70, 126, and 266. | |
$70Hint: Not a factor of 126 or 266, so couldn't be correct. |
Question 44 |
How many factors does 80 have?
\( \large8\) Hint: Don't forget 1 and 80. | |
\( \large9\) Hint: Only perfect squares have an odd number of factors -- otherwise factors come in pairs. | |
\( \large10\) Hint: 1,2,4,5,8,10,16,20,40,80 | |
\( \large12\) Hint: Did you count a number twice? Include a number that isn't a factor? |
Question 45 |
The student used a method that worked for this problem and can be generalized to any subtraction problem.Hint: Note that this algorithm is taught as the "standard" algorithm in much of Europe (it's where the term "borrowing" came from -- you borrow on top and "pay back" on the bottom). | |
The student used a method that worked for this problem and that will work for any subtraction problem that only requires one regrouping; it will not work if more regrouping is required.Hint: Try some more examples. | |
The student used a method that worked for this problem and will work for all three-digit subtraction problems, but will not work for larger problems.Hint: Try some more examples. | |
The student used a method that does not work. The student made two mistakes that cancelled each other out and was lucky to get the right answer for this problem.Hint: Remember, there are many ways to do subtraction; there is no one "right" algorithm. |
|
List |
If you found a mistake or have comments on a particular question, please contact me (please copy and paste at least part of the question into the form, as the numbers change depending on how quizzes are displayed). General comments can be left here.