Hints will display for most wrong answers; explanations for most right answers.   You can attempt a question multiple times; it will only be scored correct if you get it right the first time.  To see five new questions, reload the page.

I used the official objectives and sample test to construct these questions, but cannot promise that they accurately reflect what’s on the real test.   Some of the sample questions were more convoluted than I could bear to write.   See terms of use.   See the MTEL Practice Test main page to view questions on a particular topic or to download paper practice tests.

MTEL General Curriculum Mathematics Practice

Question 1

Taxicab fares in Boston (Spring 2012) are $2.60 for the first \(\dfrac{1}{7}\) of a mile or less and $0.40 for each \(\dfrac{1}{7}\) of a mile after that.

Let d represent the distance a passenger travels in miles (with \(d>\dfrac{1}{7}\)). Which of the following expressions represents the total fare?

A
\( \large \$2.60+\$0.40d\)
Hint:
It's 40 cents for 1/7 of a mile, not per mile.
B
\( \large \$2.60+\$0.40\dfrac{d}{7}\)
Hint:
According to this equation, going 7 miles would cost $3; does that make sense?
C
\( \large \$2.20+\$2.80d\)
Hint:
You can think of the fare as $2.20 to enter the cab, and then $0.40 for each 1/7 of a mile, including the first 1/7 of a mile (or $2.80 per mile).

Alternatively, you pay $2.60 for the first 1/7 of a mile, and then $2.80 per mile for d-1/7 miles. The total is 2.60+2.80(d-1/7) = 2.60+ 2.80d -.40 = 2.20+2.80d.
D
\( \large \$2.60+\$2.80d\)
Hint:
Don't count the first 1/7 of a mile twice.
Question 1 Explanation: 
Topic: Identify variables and derive algebraic expressions that represent real-world situations (Objective 0020), and select the linear equation that best models a real-world situation (Objective 0022).
Question 2

Use the problem below to answer the question that follows:

T shirts are on sale for 20% off. Tasha paid $8.73 for a shirt.  What is the regular price of the shirt? There is no tax on clothing purchases under $175.

Let p represent the regular price of these t-shirt. Which of the following equations is correct?

A
\( \large 0.8p=\$8.73\)
Hint:
80% of the regular price = $8.73.
B
\( \large \$8.73+0.2*\$8.73=p\)
Hint:
The 20% off was off of the ORIGINAL price, not off the $8.73 (a lot of people make this mistake). Plus this is the same equation as in choice c.
C
\( \large 1.2*\$8.73=p\)
Hint:
The 20% off was off of the ORIGINAL price, not off the $8.73 (a lot of people make this mistake). Plus this is the same equation as in choice b.
D
\( \large p-0.2*\$8.73=p\)
Hint:
Subtract p from both sides of this equation, and you have -.2 x 8.73 =0.
Question 2 Explanation: 
Topics: Use algebra to solve word problems involving percents and identify variables, and derive algebraic expressions that represent real-world situations (Objective 0020).
Question 3

Here is a number trick:

 1) Pick a whole number

 2) Double your number.

 3) Add 20 to the above result.

 4) Multiply the above by 5

 5) Subtract 100

 6) Divide by 10

The result is always the number that you started with! Suppose you start by picking N. Which of the equations below best demonstrates that the result after Step 6 is also N?

A
\( \large N*2+20*5-100\div 10=N\)
Hint:
Use parentheses or else order of operations is off.
B
\( \large \left( \left( 2*N+20 \right)*5-100 \right)\div 10=N\)
C
\( \large \left( N+N+20 \right)*5-100\div 10=N\)
Hint:
With this answer you would subtract 10, instead of subtracting 100 and then dividing by 10.
D
\( \large \left( \left( \left( N\div 10 \right)-100 \right)*5+20 \right)*2=N\)
Hint:
This answer is quite backwards.
Question 3 Explanation: 
Topic: Recognize and apply the concepts of variable, function, equality, and equation to express relationships algebraically (Objective 0020).
Question 4

The first histogram shows the average life expectancies for women in different countries in Africa in 1998; the second histogram gives similar data for Europe:

  

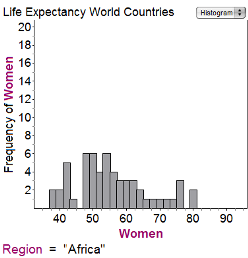
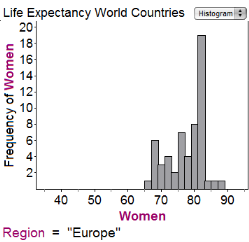
How much bigger is the range of the data for Africa than the range of the data for Europe?

A

0 years

Hint:
Range is the maximum life expectancy minus the minimum life expectancy.
B

12 years

Hint:
Are you subtracting frequencies? Range is about values of the data, not frequency.
C

18 years

Hint:
It's a little hard to read the graph, but it doesn't matter if you're consistent. It looks like the range for Africa is 80-38= 42 years and for Europe is 88-64 = 24; 42-24=18.
D

42 years

Hint:
Read the question more carefully.
Question 4 Explanation: 
Topic: Compare different data sets (Objective 0025).
Question 5

Below is a portion of a number line:

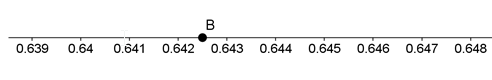
 Point B is halfway between two tick marks.  What number is represented by Point B?

 
A
\( \large 0.645\)
Hint:
That point is marked on the line, to the right.
B
\( \large 0.6421\)
Hint:
That point is to the left of point B.
C
\( \large 0.6422\)
Hint:
That point is to the left of point B.
D
\( \large 0.6425\)
Question 5 Explanation: 
Topic: Using Number Lines (Objective 0017)
There are 5 questions to complete.

If you found a mistake or have comments on a particular question, please contact me (please copy and paste at least part of the question into the form, as the numbers change depending on how quizzes are displayed).   General comments can be left here.