Hints will display for most wrong answers; explanations for most right answers. You can attempt a question multiple times; it will only be scored correct if you get it right the first time. To see ten new questions, reload the page.
I used the official objectives and sample test to construct these questions, but cannot promise that they accurately reflect what’s on the real test. Some of the sample questions were more convoluted than I could bear to write. See terms of use. See the MTEL Practice Test main page to view questions on a particular topic or to download paper practice tests.
MTEL General Curriculum Mathematics Practice
Question 1 |
The picture below shows identical circles drawn on a piece of paper. The rectangle represents an index card that is blocking your view of \( \dfrac{3}{5}\) of the circles on the paper. How many circles are covered by the rectangle?

4Hint: The card blocks more than half of the circles, so this number is too small. | |
5Hint: The card blocks more than half of the circles, so this number is too small. | |
8Hint: The card blocks more than half of the circles, so this number is too small. | |
12Hint: 2/5 of the circles or 8 circles are showing. Thus 4 circles represent 1/5 of the circles, and \(4 \times 5=20\) circles represent 5/5 or all the circles. Thus 12 circles are hidden. |
Question 2 |
Which of the lists below is in order from least to greatest value?
\( \large -0.044,\quad -0.04,\quad 0.04,\quad 0.044\) Hint: These are easier to compare if you add trailing zeroes (this is finding a common denominator) -- all in thousandths, -0.044, -0.040,0 .040, 0.044. The middle two numbers, -0.040 and 0.040 can be modeled as owing 4 cents and having 4 cents. The outer two numbers are owing or having a bit more. | |
\( \large -0.04,\quad -0.044,\quad 0.044,\quad 0.04\) Hint: 0.04=0.040, which is less than 0.044. | |
\( \large -0.04,\quad -0.044,\quad 0.04,\quad 0.044\) Hint: -0.04=-0.040, which is greater than \(-0.044\). | |
\( \large -0.044,\quad -0.04,\quad 0.044,\quad 0.04\) Hint: 0.04=0.040, which is less than 0.044. |
Question 3 |
The prime factorization of n can be written as n=pqr, where p, q, and r are distinct prime numbers. How many factors does n have, including 1 and itself?
\( \large3\) Hint: 1, p, q, r, and pqr are already 5, so this isn't enough. You might try plugging in p=2, q=3, and r=5 to help with this problem. | |
\( \large5\) Hint: Don't forget pq, etc. You might try plugging in p=2, q=3, and r=5 to help with this problem. | |
\( \large6\) Hint: You might try plugging in p=2, q=3, and r=5 to help with this problem. | |
\( \large8\) Hint: 1, p, q, r, pq, pr, qr, pqr. |
Question 4 |
Taxicab fares in Boston (Spring 2012) are $2.60 for the first \(\dfrac{1}{7}\) of a mile or less and $0.40 for each \(\dfrac{1}{7}\) of a mile after that.
Let d represent the distance a passenger travels in miles (with \(d>\dfrac{1}{7}\)). Which of the following expressions represents the total fare?
\( \large \$2.60+\$0.40d\) Hint: It's 40 cents for 1/7 of a mile, not per mile. | |
\( \large \$2.60+\$0.40\dfrac{d}{7}\) Hint: According to this equation, going 7 miles would cost $3; does that make sense? | |
\( \large \$2.20+\$2.80d\) Hint: You can think of the fare as $2.20 to enter the cab, and then $0.40 for each 1/7 of a mile, including the first 1/7 of a mile (or $2.80 per mile).
Alternatively, you pay $2.60 for the first 1/7 of a mile, and then $2.80 per mile for d-1/7 miles. The total is 2.60+2.80(d-1/7) = 2.60+ 2.80d -.40 = 2.20+2.80d. | |
\( \large \$2.60+\$2.80d\) Hint: Don't count the first 1/7 of a mile twice. |
Question 5 |
What is the probability that two randomly selected people were born on the same day of the week? Assume that all days are equally probable.
\( \large \dfrac{1}{7}\) Hint: It doesn't matter what day the first person was born on. The probability that the second person will match is 1/7 (just designate one person the first and the other the second). Another way to look at it is that if you list the sample space of all possible pairs, e.g. (Wed, Sun), there are 49 such pairs, and 7 of them are repeats of the same day, and 7/49=1/7. | |
\( \large \dfrac{1}{14}\) Hint: What would be the sample space here? Ie, how would you list 14 things that you pick one from? | |
\( \large \dfrac{1}{42}\) Hint: If you wrote the seven days of the week on pieces of paper and put the papers in a jar, this would be the probability that the first person picked Sunday and the second picked Monday from the jar -- not the same situation. | |
\( \large \dfrac{1}{49}\) Hint: This is the probability that they are both born on a particular day, e.g. Sunday. |
Question 6 |
The first histogram shows the average life expectancies for women in different countries in Africa in 1998; the second histogram gives similar data for Europe:
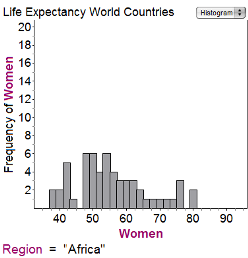
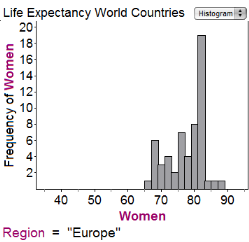
How much bigger is the range of the data for Africa than the range of the data for Europe?
0 yearsHint: Range is the maximum life expectancy minus the minimum life expectancy. | |
12 yearsHint: Are you subtracting frequencies? Range is about values of the data, not frequency. | |
18 yearsHint: It's a little hard to read the graph, but it doesn't matter if you're consistent. It looks like the range for Africa is 80-38= 42 years and for Europe is 88-64 = 24; 42-24=18. | |
42 yearsHint: Read the question more carefully. |
Question 7 |
Four children randomly line up, single file. What is the probability that they are in height order, with the shortest child in front? All of the children are different heights.
\( \large \dfrac{1}{4}\) Hint: Try a simpler question with 3 children -- call them big, medium, and small -- and list all the ways they could line up. Then see how to extend your logic to the problem with 4 children. | |
\( \large \dfrac{1}{256}
\) Hint: Try a simpler question with 3 children -- call them big, medium, and small -- and list all the ways they could line up. Then see how to extend your logic to the problem with 4 children. | |
\( \large \dfrac{1}{16}\) Hint: Try a simpler question with 3 children -- call them big, medium, and small -- and list all the ways they could line up. Then see how to extend your logic to the problem with 4 children. | |
\( \large \dfrac{1}{24}\) Hint: The number of ways for the children to line up is \(4!=4 \times 3 \times 2 \times 1 =24\) -- there are 4 choices for who is first in line, then 3 for who is second, etc. Only one of these lines has the children in the order specified. |
Question 8 |
The chairs in a large room can be arranged in rows of 18, 25, or 60 with no chairs left over. If C is the smallest possible number of chairs in the room, which of the following inequalities does C satisfy?
\( \large C\le 300\) Hint: Find the LCM. | |
\( \large 300 < C \le 500 \) Hint: Find the LCM. | |
\( \large 500 < C \le 700 \) Hint: Find the LCM. | |
\( \large C>700\) Hint: The LCM is 900, which is the smallest number of chairs. |
Question 9 |
What is the greatest common factor of 540 and 216?
\( \large{{2}^{2}}\cdot {{3}^{3}}\) Hint: One way to solve this is to factor both numbers: \(540=2^2 \cdot 3^3 \cdot 5\) and \(216=2^3 \cdot 3^3\). Then take the smaller power for each prime that is a factor of both numbers. | |
\( \large2\cdot 3\) Hint: This is a common factor of both numbers, but it's not the greatest common factor. | |
\( \large{{2}^{3}}\cdot {{3}^{3}}\) Hint: \(2^3 = 8\) is not a factor of 540. | |
\( \large{{2}^{2}}\cdot {{3}^{2}}\) Hint: This is a common factor of both numbers, but it's not the greatest common factor. |
Question 10 |
In March of 2012, 1 dollar was worth the same as 0.761 Euros, and 1 dollar was also worth the same as 83.03 Japanese Yen. Which of the expressions below gives the number of Yen that are worth 1 Euro?
\( \large {83}.0{3}\cdot 0.{761}\) Hint: This equation gives less than the number of yen per dollar, but 1 Euro is worth more than 1 dollar. | |
\( \large \dfrac{0.{761}}{{83}.0{3}}\) Hint: Number is way too small. | |
\( \large \dfrac{{83}.0{3}}{0.{761}}\) Hint: One strategy here is to use easier numbers, say 1 dollar = .5 Euros and 100 yen, then 1 Euro would be 200 Yen (change the numbers in the equations and see what works). Another is to use dimensional analysis: we want # yen per Euro, or yen/Euro = yen/dollar \(\times\) dollar/Euro = \(83.03 \times \dfrac {1}{0.761}\) | |
\( \large \dfrac{1}{0.{761}}\cdot \dfrac{1}{{83}.0{3}}\) Hint: Number is way too small. |
If you found a mistake or have comments on a particular question, please contact me (please copy and paste at least part of the question into the form, as the numbers change depending on how quizzes are displayed). General comments can be left here.