Hints will display for most wrong answers; explanations for most right answers. You can attempt a question multiple times; it will only be scored correct if you get it right the first time.
I used the official objectives and sample test to construct these questions, but cannot promise that they accurately reflect what’s on the real test. Some of the sample questions were more convoluted than I could bear to write. See terms of use. See the MTEL Practice Test main page to view questions on a particular topic or to download paper practice tests.
MTEL General Curriculum Mathematics Practice
Question 1 |
In March of 2012, 1 dollar was worth the same as 0.761 Euros, and 1 dollar was also worth the same as 83.03 Japanese Yen. Which of the expressions below gives the number of Yen that are worth 1 Euro?
\( \large {83}.0{3}\cdot 0.{761}\) Hint: This equation gives less than the number of yen per dollar, but 1 Euro is worth more than 1 dollar. | |
\( \large \dfrac{0.{761}}{{83}.0{3}}\) Hint: Number is way too small. | |
\( \large \dfrac{{83}.0{3}}{0.{761}}\) Hint: One strategy here is to use easier numbers, say 1 dollar = .5 Euros and 100 yen, then 1 Euro would be 200 Yen (change the numbers in the equations and see what works). Another is to use dimensional analysis: we want # yen per Euro, or yen/Euro = yen/dollar \(\times\) dollar/Euro = \(83.03 \times \dfrac {1}{0.761}\) | |
\( \large \dfrac{1}{0.{761}}\cdot \dfrac{1}{{83}.0{3}}\) Hint: Number is way too small. |
Question 2 |
Kendra is trying to decide which fraction is greater, \( \dfrac{4}{7}\) or \( \dfrac{5}{8}\). Which of the following answers shows the best reasoning?
\( \dfrac{4}{7}\) is \( \dfrac{3}{7}\)away from 1, and \( \dfrac{5}{8}\) is \( \dfrac{3}{8}\)away from 1. Since eighth‘s are smaller than seventh‘s, \( \dfrac{5}{8}\) is closer to 1, and is the greater of the two fractions. | |
\( 7-4=3\) and \( 8-5=3\), so the fractions are equal.Hint: Not how to compare fractions. By this logic, 1/2 and 3/4 are equal, but 1/2 and 2/4 are not. | |
\( 4\times 8=32\) and \( 7\times 5=35\). Since \( 32<35\) , \( \dfrac{5}{8}<\dfrac{4}{7}\)Hint: Starts out as something that works, but the conclusion is wrong. 4/7 = 32/56 and 5/8 = 35/56. The cross multiplication gives the numerators, and 35/56 is bigger. | |
\( 4<5\) and \( 7<8\), so \( \dfrac{4}{7}<\dfrac{5}{8}\)Hint: Conclusion is correct, logic is wrong. With this reasoning, 1/2 would be less than 2/100,000. |
Question 3 |
The "houses" below are made of toothpicks and gum drops.
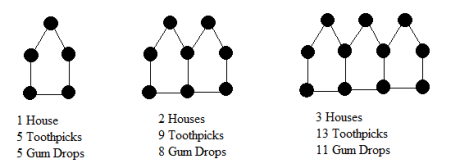
How many toothpicks are there in a row of 53 houses?
212Hint: Can the number of toothpicks be even? | |
213Hint: One way to see this is that every new "house" adds 4 toothpicks to the leftmost vertical toothpick -- so the total number is 1 plus 4 times the number of "houses." There are many other ways to look at the problem too. | |
217Hint: Try your strategy with a smaller number of "houses" so you can count and find your mistake. | |
265Hint: Remember that the "houses" overlap some walls. |
Question 4 |
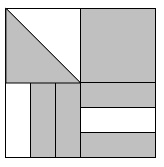
\( \large \dfrac{17}{24}\) Hint: You might try adding segments so each quadrant is divided into 6 pieces with equal area -- there will be 24 regions, not all the same shape, but all the same area, with 17 of them shaded (for the top left quarter, you could also first change the diagonal line to a horizontal or vertical line that divides the square in two equal pieces and shade one) . | |
\( \large \dfrac{3}{4}\) Hint: Be sure you're taking into account the different sizes of the pieces. | |
\( \large \dfrac{2}{3}\) Hint: The bottom half of the picture is 2/3 shaded, and the top half is more than 2/3 shaded, so this answer is too small. | |
\( \large \dfrac{17}{6} \) Hint: This answer is bigger than 1, so doesn't make any sense. Be sure you are using the whole picture, not one quadrant, as the unit. |
Question 5 |
Four children randomly line up, single file. What is the probability that they are in height order, with the shortest child in front? All of the children are different heights.
\( \large \dfrac{1}{4}\) Hint: Try a simpler question with 3 children -- call them big, medium, and small -- and list all the ways they could line up. Then see how to extend your logic to the problem with 4 children. | |
\( \large \dfrac{1}{256}
\) Hint: Try a simpler question with 3 children -- call them big, medium, and small -- and list all the ways they could line up. Then see how to extend your logic to the problem with 4 children. | |
\( \large \dfrac{1}{16}\) Hint: Try a simpler question with 3 children -- call them big, medium, and small -- and list all the ways they could line up. Then see how to extend your logic to the problem with 4 children. | |
\( \large \dfrac{1}{24}\) Hint: The number of ways for the children to line up is \(4!=4 \times 3 \times 2 \times 1 =24\) -- there are 4 choices for who is first in line, then 3 for who is second, etc. Only one of these lines has the children in the order specified. |
Question 6 |
A family went on a long car trip. Below is a graph of how far they had driven at each hour.
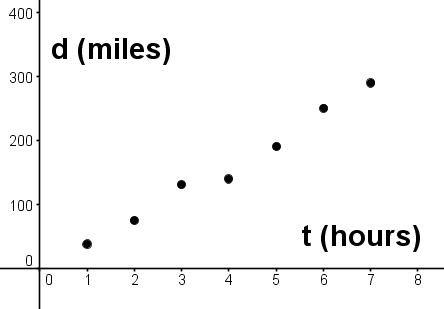
Which of the following is closest to their average speed driving on the trip?
\( \large d=20t\) Hint: Try plugging t=7 into the equation, and see how it matches the graph. | |
\( \large d=30t\) Hint: Try plugging t=7 into the equation, and see how it matches the graph. | |
\( \large d=40t\) | |
\( \large d=50t\) Hint: Try plugging t=7 into the equation, and see how it matches the graph. |
Question 7 |
Use the four figures below to answer the question that follows:

How many of the figures pictured above have at least one line of reflective symmetry?
\( \large 1\) | |
\( \large 2\) Hint: The ellipse has 2 lines of reflective symmetry (horizontal and vertical, through the center) and the triangle has 3. The other two figures have rotational symmetry, but not reflective symmetry. | |
\( \large 3\) | |
\( \large 4\) Hint: All four have rotational symmetry, but not reflective symmetry. |
Question 8 |
Which of the lines depicted below is a graph of \( \large y=2x-5\)?
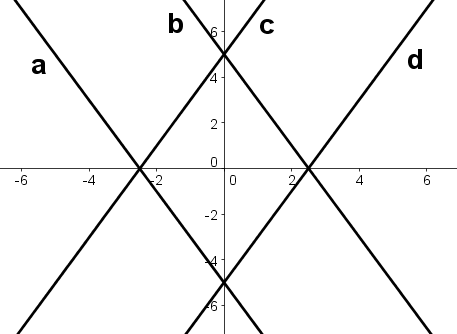
aHint: The slope of line a is negative. | |
bHint: Wrong slope and wrong intercept. | |
cHint: The intercept of line c is positive. | |
dHint: Slope is 2 -- for every increase of 1 in x, y increases by 2. Intercept is -5 -- the point (0,-5) is on the line. |
Question 9 |
Use the expression below to answer the question that follows.
\( \large \dfrac{\left( 4\times {{10}^{3}} \right)\times \left( 3\times {{10}^{4}} \right)}{6\times {{10}^{6}}}\)
Which of the following is equivalent to the expression above?
2Hint: \(10^3 \times 10^4=10^7\), and note that if you're guessing when the answers are so closely related, you're generally better off guessing one of the middle numbers. | |
20Hint: \( \dfrac{\left( 4\times {{10}^{3}} \right)\times \left( 3\times {{10}^{4}} \right)}{6\times {{10}^{6}}}=\dfrac {12 \times {{10}^{7}}}{6\times {{10}^{6}}}=\)\(2 \times {{10}^{1}}=20 \) | |
200Hint: \(10^3 \times 10^4=10^7\) | |
2000Hint: \(10^3 \times 10^4=10^7\), and note that if you're guessing when the answers are so closely related, you're generally better off guessing one of the middle numbers. |
Question 10 |
Which of the numbers below is a fraction equivalent to \( 0.\bar{6}\)?
\( \large \dfrac{4}{6}\) Hint: \( 0.\bar{6}=\dfrac{2}{3}=\dfrac{4}{6}\) | |
\( \large \dfrac{3}{5}\) Hint: This is equal to 0.6, without the repeating decimal. Answer is equivalent to choice c, which is another way to tell that it's wrong. | |
\( \large \dfrac{6}{10}\) Hint: This is equal to 0.6, without the repeating decimal. Answer is equivalent to choice b, which is another way to tell that it's wrong. | |
\( \large \dfrac{1}{6}\) Hint: This is less than a half, and \( 0.\bar{6}\) is greater than a half. |
Question 11 |
M is a multiple of 26. Which of the following cannot be true?
M is odd.Hint: All multiples of 26 are also multiples of 2, so they must be even. | |
M is a multiple of 3.Hint: 3 x 26 is a multiple of both 3 and 26. | |
M is 26.Hint: 1 x 26 is a multiple of 26. | |
M is 0.Hint: 0 x 26 is a multiple of 26. |
Question 12 |
The first histogram shows the average life expectancies for women in different countries in Africa in 1998; the second histogram gives similar data for Europe:
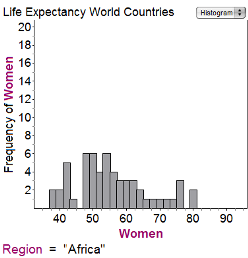
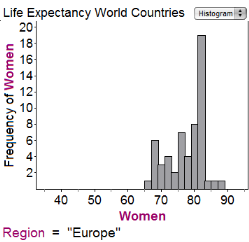
How much bigger is the range of the data for Africa than the range of the data for Europe?
0 yearsHint: Range is the maximum life expectancy minus the minimum life expectancy. | |
12 yearsHint: Are you subtracting frequencies? Range is about values of the data, not frequency. | |
18 yearsHint: It's a little hard to read the graph, but it doesn't matter if you're consistent. It looks like the range for Africa is 80-38= 42 years and for Europe is 88-64 = 24; 42-24=18. | |
42 yearsHint: Read the question more carefully. |
Question 13 |
Which of the numbers below is not equivalent to 4%?
\( \large \dfrac{1}{25}\) Hint: 1/25=4/100, so this is equal to 4% (be sure you read the question correctly). | |
\( \large \dfrac{4}{100}\) Hint: 4/100=4% (be sure you read the question correctly). | |
\( \large 0.4\) Hint: 0.4=40% so this is not equal to 4% | |
\( \large 0.04\) Hint: 0.04=4/100, so this is equal to 4% (be sure you read the question correctly). |
Question 14 |
Which property is not shared by all rhombi?
4 congruent sidesHint: The most common definition of a rhombus is a quadrilateral with 4 congruent sides. | |
A center of rotational symmetryHint: The diagonal of a rhombus separates it into two congruent isosceles triangles. The center of this line is a center of 180 degree rotational symmetry that switches the triangles. | |
4 congruent anglesHint: Unless the rhombus is a square, it does not have 4 congruent angles. | |
2 sets of parallel sidesHint: All rhombi are parallelograms. |
Question 15 |
Which of the lists below contains only irrational numbers?
\( \large\pi , \quad \sqrt{6},\quad \sqrt{\dfrac{1}{2}}\) | |
\( \large\pi , \quad \sqrt{9}, \quad \pi +1\) Hint: \( \sqrt{9}=3\) | |
\( \large\dfrac{1}{3},\quad \dfrac{5}{4},\quad \dfrac{2}{9}\) Hint: These are all rational. | |
\( \large-3,\quad 14,\quad 0\) Hint: These are all rational. |
Question 16 |
What is the length of side \(\overline{BD}\) in the triangle below, where \(\angle DBA\) is a right angle?
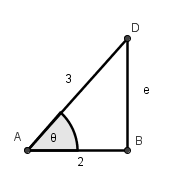
\( \large 1\) Hint: Use the Pythagorean Theorem. | |
\( \large \sqrt{5}\) Hint: \(2^2+e^2=3^2\) or \(4+e^2=9;e^2=5; e=\sqrt{5}\). | |
\( \large \sqrt{13}\) Hint: e is not the hypotenuse. | |
\( \large 5\) Hint: Use the Pythagorean Theorem. |
Question 17 |
Use the expression below to answer the question that follows.
\( \large 3\times {{10}^{4}}+2.2\times {{10}^{2}}\)
Which of the following is closest to the expression above?
Five millionHint: Pay attention to the exponents. Adding 3 and 2 doesn't work because they have different place values. | |
Fifty thousandHint: Pay attention to the exponents. Adding 3 and 2 doesn't work because they have different place values. | |
Three millionHint: Don't add the exponents. | |
Thirty thousandHint: \( 3\times {{10}^{4}} = 30,000;\) the other term is much smaller and doesn't change the estimate. |
Question 18 |
Which of the following is equivalent to \( \dfrac{3}{4}-\dfrac{1}{8}+\dfrac{2}{8}\times \dfrac{1}{2}?\)
\( \large \dfrac{7}{16}\) Hint: Multiplication comes before addition and subtraction in the order of operations. | |
\( \large \dfrac{1}{2}\) Hint: Addition and subtraction are of equal priority in the order of operations -- do them left to right. | |
\( \large \dfrac{3}{4}\) Hint: \( \dfrac{3}{4}-\dfrac{1}{8}+\dfrac{2}{8}\times \dfrac{1}{2}\)=\( \dfrac{3}{4}-\dfrac{1}{8}+\dfrac{1}{8}\)=\( \dfrac{3}{4}+-\dfrac{1}{8}+\dfrac{1}{8}\)=\( \dfrac{3}{4}\) | |
\( \large \dfrac{3}{16}\) Hint: Multiplication comes before addition and subtraction in the order of operations. |
Question 19 |
Use the solution procedure below to answer the question that follows:
\( \large {\left( x+3 \right)}^{2}=10\)
\( \large \left( x+3 \right)\left( x+3 \right)=10\)
\( \large {x}^{2}+9=10\)
\( \large {x}^{2}+9-9=10-9\)
\( \large {x}^{2}=1\)
\( \large x=1\text{ or }x=-1\)
Which of the following is incorrect in the procedure shown above?
The commutative property is used incorrectly.Hint: The commutative property is \(a+b=b+a\) or \(ab=ba\). | |
The associative property is used incorrectly.Hint: The associative property is \(a+(b+c)=(a+b)+c\) or
\(a \times (b \times c)=(a \times b) \times c\). | |
Order of operations is done incorrectly. | |
The distributive property is used incorrectly.Hint: \((x+3)(x+3)=x(x+3)+3(x+3)\)=\(x^2+3x+3x+9.\) |
Question 20 |
Here is a student's work solving an equation:
\( x-4=-2x+6\)
\( x-4+4=-2x+6+4\)
\( x=-2x+10\)
\( x-2x=10\)
\( x=10\)
Which of the following statements is true?
The student‘s solution is correct.Hint: Try plugging into the original solution. | |
The student did not correctly use properties of equality.Hint: After \( x=-2x+10\), the student subtracted 2x on the left and added 2x on the right. | |
The student did not correctly use the distributive property.Hint: Distributive property is \(a(b+c)=ab+ac\). | |
The student did not correctly use the commutative property.Hint: Commutative property is \(a+b=b+a\) or \(ab=ba\). |
Question 21 |
The pattern below consists of a row of black squares surrounded by white squares.
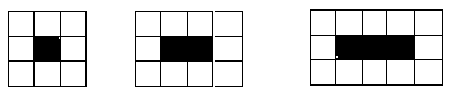
How many white squares would surround a row of 157 black squares?
314Hint: Try your procedure on a smaller number that you can count to see where you made a mistake. | |
317Hint: Are there ever an odd number of white squares? | |
320Hint: One way to see this is that there are 6 tiles on the left and right ends, and the rest of the white tiles are twice the number of black tiles (there are many other ways to look at it too). | |
322Hint: Try your procedure on a smaller number that you can count to see where you made a mistake. |
Question 22 |
Use the expression below to answer the question that follows.
\(\large \dfrac{\left( 155 \right)\times \left( 6,124 \right)}{977}\)
Which of the following is the best estimate of the expression above?
100Hint: 6124/977 is approximately 6. | |
200Hint: 6124/977 is approximately 6. | |
1,000Hint: 6124/977 is approximately 6. 155 is approximately 150, and \( 6 \times 150 = 3 \times 300 = 900\), so this answer is closest. | |
2,000Hint: 6124/977 is approximately 6. |
Question 23 |
The prime factorization of n can be written as n=pqr, where p, q, and r are distinct prime numbers. How many factors does n have, including 1 and itself?
\( \large3\) Hint: 1, p, q, r, and pqr are already 5, so this isn't enough. You might try plugging in p=2, q=3, and r=5 to help with this problem. | |
\( \large5\) Hint: Don't forget pq, etc. You might try plugging in p=2, q=3, and r=5 to help with this problem. | |
\( \large6\) Hint: You might try plugging in p=2, q=3, and r=5 to help with this problem. | |
\( \large8\) Hint: 1, p, q, r, pq, pr, qr, pqr. |
Question 24 |
Below is a portion of a number line.
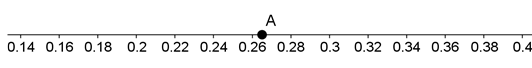
Point A is one-quarter of the distance from 0.26 to 0.28. What number is represented by point A?
\( \large0.26\) Hint: Please reread the question. | |
\( \large0.2625\) Hint: This is one-quarter of the distance between 0.26 and 0.27, which is not what the question asked. | |
\( \large0.265\) | |
\( \large0.27\) Hint: Please read the question more carefully. This answer would be correct if Point A were halfway between the tick marks, but it's not. |
Question 25 |
The letters A, B, and C represent digits (possibly equal) in the twelve digit number x=111,111,111,ABC. For which values of A, B, and C is x divisible by 40?
\( \large A = 3, B = 2, C=0\) Hint: Note that it doesn't matter what the first 9 digits are, since 1000 is divisible by 40, so DEF,GHI,JKL,000 is divisible by 40 - we need to check the last 3. | |
\( \large A = 0, B = 0, C=4\) Hint: Not divisible by 10, since it doesn't end in 0. | |
\( \large A = 4, B = 2, C=0\) Hint: Divisible by 10 and by 4, but not by 40, as it's not divisible by 8. Look at 40 as the product of powers of primes -- 8 x 5, and check each. To check 8, either check whether 420 is divisible by 8, or take ones place + twice tens place + 4 * hundreds place = 18, which is not divisible by 8. | |
\( \large A =1, B=0, C=0\) Hint: Divisible by 10 and by 4, but not by 40, as it's not divisible by 8. Look at 40 as the product of powers of primes -- 8 x 5, and check each. To check 8, either check whether 100 is divisible by 8, or take ones place + twice tens place + 4 * hundreds place = 4, which is not divisible by 8. |
Question 26 |
Which of the following inequalities describes all values of x with \(\large \dfrac{x}{2}\le \dfrac{x}{3}\)?
\( \large x < 0\) Hint: If x =0, then x/2 = x/3, so this answer can't be correct. | |
\( \large x \le 0\) | |
\( \large x > 0\) Hint: If x =0, then x/2 = x/3, so this answer can't be correct. | |
\( \large x \ge 0\) Hint: Try plugging in x = 6. |
Question 27 |
If x is an integer, which of the following must also be an integer?
\( \large \dfrac{x}{2}\) Hint: If x is odd, then \( \dfrac{x}{2} \) is not an integer, e.g. 3/2 = 1.5. | |
\( \large \dfrac{2}{x}\) Hint: Only an integer if x = -2, -1, 1, or 2. | |
\( \large-x\) Hint: -1 times any integer is still an integer. | |
\(\large\sqrt{x}\) Hint: Usually not an integer, e.g. \( \sqrt{2} \approx 1.414 \). |
Question 28 |
Given that 10 cm is approximately equal to 4 inches, which of the following expressions models a way to find out approximately how many inches are equivalent to 350 cm?
\( \large 350\times \left( \dfrac{10}{4} \right)\) Hint: The final result should be smaller than 350, and this answer is bigger. | |
\( \large 350\times \left( \dfrac{4}{10} \right)\) Hint: Dimensional analysis can help here: \(350 \text{cm} \times \dfrac{4 \text{in}}{10 \text{cm}}\). The cm's cancel and the answer is in inches. | |
\( \large (10-4) \times 350
\) Hint: This answer doesn't make much sense. Try with a simpler example (e.g. 20 cm not 350 cm) to make sure that your logic makes sense. | |
\( \large (350-10) \times 4\) Hint: This answer doesn't make much sense. Try with a simpler example (e.g. 20 cm not 350 cm) to make sure that your logic makes sense. |
Question 29 |
Which of the following is not possible?
An equiangular triangle that is not equilateral.Hint: The AAA property of triangles states that all triangles with corresponding angles congruent are similar. Thus all triangles with three equal angles are similar, and are equilateral. | |
An equiangular quadrilateral that is not equilateral.Hint: A rectangle is equiangular (all angles the same measure), but if it's not a square, it's not equilateral (all sides the same length). | |
An equilateral quadrilateral that is not equiangular.Hint: This rhombus has equal sides, but it doesn't have equal angles:  | |
An equiangular hexagon that is not equilateral.Hint: This hexagon has equal angles, but it doesn't have equal sides: 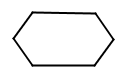 |
Question 30 |
The histogram below shows the number of pairs of footware owned by a group of college students.
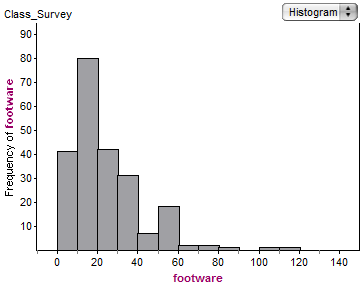
Which of the following statements can be inferred from the graph above?
The median number of pairs of footware owned is between 50 and 60 pairs.Hint: The same number of data points are less than the median as are greater than the median -- but on this histogram, clearly more than half the students own less than 50 pairs of shoes, so the median is less than 50. | |
The mode of the number of pairs of footware owned is 20.Hint: The mode is the most common number of pairs of footwear owned. We can't tell it from this histogram because each bar represents 10 different numbers-- perhaps 8 students each own each number from 10 to 19, but 40 students own exactly 6 pairs of shoes.... or perhaps not.... | |
The mean number of pairs of footware owned is less than the median number of pairs of footware owned.Hint: This is a right skewed distribution, and so the mean is bigger than the median -- the few large values on the right pull up the mean, but have little effect on the median. | |
The median number of pairs of footware owned is between 10 and 20.Hint: There are approximately 230 students represented in this survey, and the 41st through 120th lowest values are between 10 and 20 -- thus the middle value is in that range. |
Question 31 |
A biology class requires a lab fee, which is a whole number of dollars, and the same amount for all students. On Monday the instructor collected $70 in fees, on Tuesday she collected $126, and on Wednesday she collected $266. What is the largest possible amount the fee could be?
$2Hint: A possible fee, but not the largest possible fee. Check the other choices to see which are factors of all three numbers. | |
$7Hint: A possible fee, but not the largest possible fee. Check the other choices to see which are factors of all three numbers. | |
$14Hint: This is the greatest common factor of 70, 126, and 266. | |
$70Hint: Not a factor of 126 or 266, so couldn't be correct. |
Question 32 |
Taxicab fares in Boston (Spring 2012) are $2.60 for the first \(\dfrac{1}{7}\) of a mile or less and $0.40 for each \(\dfrac{1}{7}\) of a mile after that.
Let d represent the distance a passenger travels in miles (with \(d>\dfrac{1}{7}\)). Which of the following expressions represents the total fare?
\( \large \$2.60+\$0.40d\) Hint: It's 40 cents for 1/7 of a mile, not per mile. | |
\( \large \$2.60+\$0.40\dfrac{d}{7}\) Hint: According to this equation, going 7 miles would cost $3; does that make sense? | |
\( \large \$2.20+\$2.80d\) Hint: You can think of the fare as $2.20 to enter the cab, and then $0.40 for each 1/7 of a mile, including the first 1/7 of a mile (or $2.80 per mile).
Alternatively, you pay $2.60 for the first 1/7 of a mile, and then $2.80 per mile for d-1/7 miles. The total is 2.60+2.80(d-1/7) = 2.60+ 2.80d -.40 = 2.20+2.80d. | |
\( \large \$2.60+\$2.80d\) Hint: Don't count the first 1/7 of a mile twice. |
Question 33 |
A solution requires 4 ml of saline for every 7 ml of medicine. How much saline would be required for 50 ml of medicine?
\( \large 28 \dfrac{4}{7}\) ml Hint: 49 ml of medicine requires 28 ml of saline. The extra ml of saline requires 4 ml saline/ 7 ml medicine = 4/7 ml saline per 1 ml medicine. | |
\( \large 28 \dfrac{1}{4}\) ml Hint: 49 ml of medicine requires 28 ml of saline. How much saline does the extra ml require? | |
\( \large 28 \dfrac{1}{7}\) ml Hint: 49 ml of medicine requires 28 ml of saline. How much saline does the extra ml require? | |
\( \large 87.5\) ml Hint: 49 ml of medicine requires 28 ml of saline. How much saline does the extra ml require? |
Question 34 |
The letters A, and B represent digits (possibly equal) in the ten digit number x=1,438,152,A3B. For which values of A and B is x divisible by 12, but not by 9?
\( \large A = 0, B = 4\) Hint: Digits add to 31, so not divisible by 3, so not divisible by 12. | |
\( \large A = 7, B = 2\) Hint: Digits add to 36, so divisible by 9. | |
\( \large A = 0, B = 6\) Hint: Digits add to 33, divisible by 3, not 9. Last digits are 36, so divisible by 4, and hence by 12. | |
\( \large A = 4, B = 8\) Hint: Digits add to 39, divisible by 3, not 9. Last digits are 38, so not divisible by 4, so not divisible by 12. |
Question 35 |
A sales companies pays its representatives $2 for each item sold, plus 40% of the price of the item. The rest of the money that the representatives collect goes to the company. All transactions are in cash, and all items cost $4 or more. If the price of an item in dollars is p, which expression represents the amount of money the company collects when the item is sold?
\( \large \dfrac{3}{5}p-2\) Hint: The company gets 3/5=60% of the price, minus the $2 per item. | |
\( \large \dfrac{3}{5}\left( p-2 \right)\) Hint: This is sensible, but not what the problem states. | |
\( \large \dfrac{2}{5}p+2\) Hint: The company pays the extra $2; it doesn't collect it. | |
\( \large \dfrac{2}{5}p-2\) Hint: This has the company getting 2/5 = 40% of the price of each item, but that's what the representative gets. |
Question 36 |
Aya and Kendra want to estimate the height of a tree. On a sunny day, Aya measures Kendra's shadow as 3 meters long, and Kendra measures the tree's shadow as 15 meters long. Kendra is 1.5 meters tall. How tall is the tree?
7.5 metersHint: Here is a picture, note that the large and small right triangles are similar:  One way to do the problem is to note that there is a dilation (scale) factor of 5 on the shadows, so there must be that factor on the heights too. Another way is to note that the shadows are twice as long as the heights. | |
22.5 metersHint: Draw a picture. | |
30 metersHint: Draw a picture. | |
45 metersHint: Draw a picture. |
Question 37 |
Which of the following values of x satisfies the inequality \( \large \left| {{(x+2)}^{3}} \right|<3?\)
\( \large x=-3\) Hint: \( \left| {{(-3+2)}^{3}} \right|\)=\( \left | {(-1)}^3 \right | \)=\( \left | -1 \right |=1 \) . | |
\( \large x=0\) Hint: \( \left| {{(0+2)}^{3}} \right|\)=\( \left | {2}^3 \right | \)=\( \left | 8 \right | \) =\( 8\) | |
\( \large x=-4\) Hint: \( \left| {{(-4+2)}^{3}} \right|\)=\( \left | {(-2)}^3 \right | \)=\( \left | -8 \right | \) =\( 8\) | |
\( \large x=1\) Hint: \( \left| {{(1+2)}^{3}} \right|\)=\( \left | {3}^3 \right | \)=\( \left | 27 \right | \) = \(27\) |
Question 38 |
There are six gumballs in a bag — two red and four green. Six children take turns picking a gumball out of the bag without looking. They do not return any gumballs to the bag. What is the probability that the first two children to pick from the bag pick the red gumballs?
\( \large \dfrac{1}{3}\) Hint: This is the probability that the first child picks a red gumball, but not that the first two children pick red gumballs. | |
\( \large \dfrac{1}{8}\) Hint: Are you adding things that you should be multiplying? | |
\( \large \dfrac{1}{9}\) Hint: This would be the probability if the gumballs were returned to the bag. | |
\( \large \dfrac{1}{15}\) Hint: The probability that the first child picks red is 2/6 = 1/3. Then there are 5 gumballs in the bag, one red, so the probability that the second child picks red is 1/5. Thus 1/5 of the time, after the first child picks red, the second does too, so the probability is 1/5 x 1/3 = 1/15. |
Question 39 |
The window glass below has the shape of a semi-circle on top of a square, where the side of the square has length x. It was cut from one piece of glass.
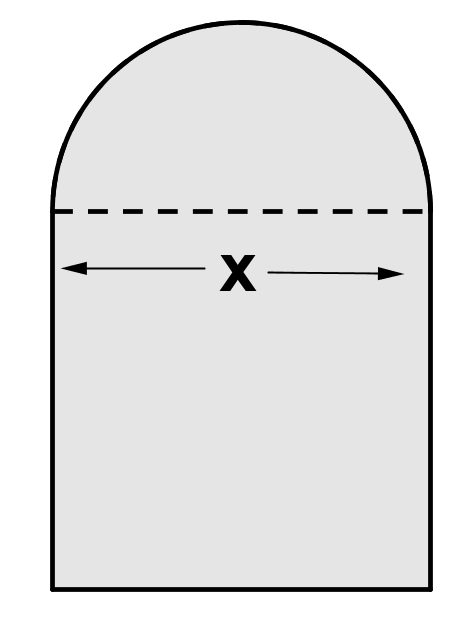
What is the perimeter of the window glass?
\( \large 3x+\dfrac{\pi x}{2}\) Hint: By definition, \(\pi\) is the ratio of the circumference of a circle to its diameter; thus the circumference is \(\pi d\). Since we have a semi-circle, its perimeter is \( \dfrac{1}{2} \pi x\). Only 3 sides of the square contribute to the perimeter. | |
\( \large 3x+2\pi x\) Hint: Make sure you know how to find the circumference of a circle. | |
\( \large 3x+\pi x\) Hint: Remember it's a semi-circle, not a circle. | |
\( \large 4x+2\pi x\) Hint: Only 3 sides of the square contribute to the perimeter. |
Question 40 |
Which of the following is closest to the height of a college student in centimeters?
1.6 cmHint: This is more the height of a Lego toy college student -- less than an inch! | |
16 cmHint: Less than knee high on most college students. | |
160 cmHint: Remember, a meter stick (a little bigger than a yard stick) is 100 cm. Also good to know is that 4 inches is approximately 10 cm. | |
1600 cmHint: This college student might be taller than some campus buildings! |
Question 41 |
Which of the following sets of polygons can be assembled to form a pentagonal pyramid?
2 pentagons and 5 rectangles.Hint: These can be assembled to form a pentagonal prism, not a pentagonal pyramid. | |
1 square and 5 equilateral triangles.Hint: You need a pentagon for a pentagonal pyramid. | |
1 pentagon and 5 isosceles triangles. | |
1 pentagon and 10 isosceles triangles. |
Question 42 |
Here is a student's work on several multiplication problems:
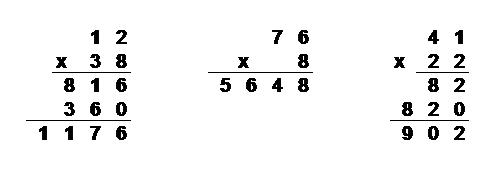 For which of the following problems is this student most likely to get the correct solution, even though he is using an incorrect algorithm?
For which of the following problems is this student most likely to get the correct solution, even though he is using an incorrect algorithm?
58 x 22Hint: This problem involves regrouping, which the student does not do correctly. | |
16 x 24Hint: This problem involves regrouping, which the student does not do correctly. | |
31 x 23Hint: There is no regrouping with this problem. | |
141 x 32Hint: This problem involves regrouping, which the student does not do correctly. |
Question 43 |
A cylindrical soup can has diameter 7 cm and height 11 cm. The can holds g grams of soup. How many grams of the same soup could a cylindrical can with diameter 14 cm and height 33 cm hold?
\( \large 6g\) Hint: You must scale in all three dimensions. | |
\( \large 12g\) Hint: Height is multiplied by 3, and diameter and radius are multiplied by 2. Since the radius is squared, final result is multiplied by \(2^2\times 3=12\). | |
\( \large 18g\) Hint: Don't square the height scale factor. | |
\( \large 36g\) Hint: Don't square the height scale factor. |
Question 44 |
What is the greatest common factor of 540 and 216?
\( \large{{2}^{2}}\cdot {{3}^{3}}\) Hint: One way to solve this is to factor both numbers: \(540=2^2 \cdot 3^3 \cdot 5\) and \(216=2^3 \cdot 3^3\). Then take the smaller power for each prime that is a factor of both numbers. | |
\( \large2\cdot 3\) Hint: This is a common factor of both numbers, but it's not the greatest common factor. | |
\( \large{{2}^{3}}\cdot {{3}^{3}}\) Hint: \(2^3 = 8\) is not a factor of 540. | |
\( \large{{2}^{2}}\cdot {{3}^{2}}\) Hint: This is a common factor of both numbers, but it's not the greatest common factor. |
Question 45 |
Some children explored the diagonals in 2 x 2 squares on pages of a calendar (where all four squares have numbers in them). They conjectured that the sum of the diagonals is always equal; in the example below, 8+16=9+15.
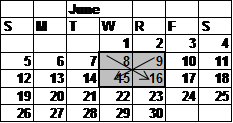
Which of the equations below could best be used to explain why the children's conjecture is correct?
\( \large 8x+16x=9x+15x\) Hint: What would x represent in this case? Make sure you can describe in words what x represents. | |
\( \large x+(x+2)=(x+1)+(x+1)\) Hint: What would x represent in this case? Make sure you can describe in words what x represents. | |
\( \large x+(x+8)=(x+1)+(x+7)\) Hint: x is the number in the top left square, x+8 is one below and to the right, x+1 is to the right of x, and x+7 is below x. | |
\( \large x+8+16=x+9+15\) Hint: What would x represent in this case? Make sure you can describe in words what x represents. |
|
List |
If you found a mistake or have comments on a particular question, please contact me (please copy and paste at least part of the question into the form, as the numbers change depending on how quizzes are displayed). General comments can be left here.